Crack growth resistance curve

The simplest case of a material's crack resistance curve would be materials which exhibit a "flat R-curve" (${\displaystyle G_{R}}$ is constant with respect to ${\displaystyle \Delta a}$). In materials with flat R-curves (the shape of R curve depends on the material properties and more importantly on the crack tip plasticity. The reduction in stress intensity factor due to high deformation can result in a flat R curve), as a crack propagates, the resistance to further crack propagation remains constant. Thus, the common failure criteria of ${\displaystyle G\geq G_{c}}$ is largely valid. In these materials, if ${\displaystyle G}$ increases as a function of ${\displaystyle \Delta a}$ (which is the case in many loading configurations and crack geometries), then as soon as the applied ${\displaystyle G}$ exceeds ${\displaystyle G_{c}}$ the crack will unstably grow to failure without ever halting.

Physically, the independence of ${\displaystyle G_{R}}$ from ${\displaystyle \Delta a}$ is indicative that in these materials the phenomena which are energetically costly during crack propagation do not evolve during crack propagation. This tends to be an accurate model for perfectly brittle materials such as ceramics, in which the principal energetic cost of fracture is the development of new free surfaces on the crack faces.[2] The character of the energetic cost of the creation of new surfaces remains largely unchanged regardless of how long the crack has propagated from its initial length.

A schematic depicting how a material's rising R-curve leads to added crack stability. Two different crack driving force curves are applied to the material, ${\displaystyle G_{1}(a)}$ and ${\displaystyle G_{2}(a)}$. Even though both are initially unstable, due to their different slopes ${\displaystyle G_{1}(a)}$ will unstably propagate the crack while ${\displaystyle G_{2}(a)}$ will arrest the crack after propagating it a short distance ${\displaystyle \delta a}$.

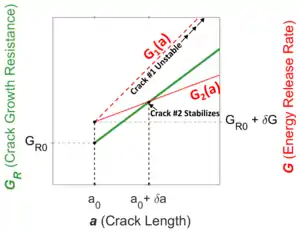
Another category of R-curve that is common in real materials is a "rising R-curve" (${\displaystyle G_{R}}$ increases as ${\displaystyle \Delta a}$ increases). In materials with rising R-curves, as a crack propagates, the resistance to further crack propagation increases, and it requires a higher and higher applied ${\displaystyle G}$ in order to achieve each subsequent increment of crack extension ${\displaystyle \delta a}$. As such, it can be technically challenging in these materials in practice to define a single value to quantify resistance to fracture (i.e. ${\displaystyle G_{c}}$ or ${\displaystyle K_{Ic}}$) as the resistance to fracture rises continuously as any given crack propagates.

Materials with rising R-curves can also more easily exhibit stable crack growth than materials with flat R-curves, even if ${\displaystyle G}$ strictly increases as a function of ${\displaystyle a}$. If at some moment in time a crack exists with initial length ${\displaystyle a_{0}}$ and an applied energy release rate which is infinitesimally exceeding the R-curve at this crack length ${\displaystyle [G(a_{0})=G_{R}(a_{0})+\delta G]}$ then this material would immediately fail if it exhibited flat R-curve behavior. If instead it exhibits rising R-curve behavior, then the crack has an added criteria for crack growth that the instantaneous slope of the driving force curve must be greater than the instantaneous slope of the crack resistance curve ${\displaystyle {\Biggl (}{\frac {\delta G(a_{0})}{\delta a}}\geq {\frac {\delta G_{R}(a_{0})}{\delta a}}{\Biggr )}}$ or else it is energetically unfavorable to grow the crack further. If ${\displaystyle G(a_{0})}$ is infinitesimally greater than ${\displaystyle G_{R}(a_{0})}$ but ${\displaystyle {\frac {\delta G(a_{0})}{\delta a}}\leq {\frac {\delta G_{R}(a_{0})}{\delta a}}}$ then the crack will grow by an infinitesimally small increment ${\displaystyle \delta a}$ such that ${\displaystyle G(a_{0}+\delta a)=G_{R}(a_{0}+\delta a)}$ and then crack growth will arrest. If the applied crack driving force ${\displaystyle G(a)}$ was gradually increased over time (through increasing the applied force for example) then this would lead to stable crack growth in this material as long as the instantaneous slope of the driving force curve continued to be less than the slope of the crack resistance curve.

Physically, the dependence of ${\displaystyle G_{R}}$ on ${\displaystyle \Delta a}$ is indicative that in rising R-curve materials, the phenomena which are energetically costly during crack propagation are evolving as the crack grows in such a way that leads to accelerated energy dissipation during crack growth. This tends to be the case in materials which undergo ductile fracture as it can be observed that the plastic zone at the crack tip increases in size as the crack propagates, indicating that an increasing amount of energy must be dissipated to plastic deformation for the crack to continue to grow.[3] A rising R-curve can also sometimes be observed in situations where a material's fracture surface becomes significantly rougher as the crack propagates, leading to additional energy dissipation as additional area of free surfaces is generated.[4]

In theory, ${\displaystyle G_{R}}$ does not continue to increase to infinity as ${\displaystyle a\rightarrow \infty }$, and instead will asymptotically approach some steady-state value after a finite amount of crack growth. It is usually not feasible to reach this steady-state condition, as it often requires very long crack extensions before reaching this condition, and thus would require large testing specimen geometries (and thus high applied forces) to observe. As such, most materials with rising R-curves are treated as if ${\displaystyle G_{R}}$ continually rises until failure.

While far less common, some materials can exhibit falling R-curves (${\displaystyle G_{R}}$ decreases as ${\displaystyle \Delta a}$ increases). In some cases, the material may initially exhibit rising R-curve behavior, reach a steady-state condition, and then transition into falling R-curve behavior. In a falling R-curve regime, as a crack propagates, the resistance to further crack propagation drops, and it requires less and less applied ${\displaystyle G}$ in order to achieve each subsequent increment of crack extension ${\displaystyle \delta a}$. Materials experiencing these conditions would exhibit highly unstable crack growth as soon as any initial crack began to propagate.

Polycrystalline graphite has been reported to demonstrate falling R-curve behavior after initially exhibiting rising R-curve behavior, which is postulated to be due to the gradual development of a microcracking damage zone in front of the crack tip which eventually dominates after the phenomena leading to the initial rising R-curve behavior reach steady-state.[5]