Crossed polygon
A crossed polygon is a polygon in the plane with a turning number or density of zero, with the appearance of a figure 8, infinity symbol, or lemniscate curve.
A crossed equilateral pentagon

The vertex figure of a snub icosidodecadodecahedron is a crossed hexagon.

A symmetric crossed decagon
Crossed polygons are related to star polygons which have turning numbers greater than 1.
The vertices with clockwise turning angles equal the vertices with counterclockwise turning angles. A crossed polygon will always have at least 2 edges or vertices intersecting or coinciding.
Any convex polygon with 4 or more sides can be remade into a crossed polygon by swapping the positions of two adjacent vertices.
Crossed polygons are common as vertex figures of uniform star polyhedra.[1]
Crossed quadrilateral
Crossed quadrilaterals are most common, including:
- crossed parallelogram or antiparallelogram, a crossed quadrilateral with alternate edges of equal length.
- crossed trapezoid' has two opposite parallel edges.
- crossed rectangle, an antiparallelogram whose edges are two opposite sides and the two diagonals of a rectangle.
- Crossed square, a crossed rectangle with two equal opposite sides and two diagonals of a square.
 Crossed square |
 Crossed trapezoid |
 Crossed parallelogram |
 Crossed rectangles |
 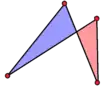 Crossed quadrilaterals |
See also
References
- Coxeter, H.S.M., M. S. Longuet-Higgins and J.C.P Miller, Uniform Polyhedra, Phil. Trans. 246 A (1954) pp. 401–450.
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.