Lift coefficient

The lift coefficient C_L is defined by[2][3]

${\displaystyle C_{\mathrm {L} }\equiv {\frac {L}{q\,S}}={\frac {L}{{\frac {1}{2}}\rho u^{2}\,S}}={\frac {2L}{\rho u^{2}S}}}$ ,

where ${\displaystyle L\,}$ is the lift force, ${\displaystyle S\,}$ is the relevant surface area and ${\displaystyle q\,}$ is the fluid dynamic pressure, in turn linked to the fluid density ${\displaystyle \rho \,}$, and to the flow speed ${\displaystyle u\,}$. The choice of the reference surface should be specified since it is arbitrary. For example, for cylindric profiles (the 3D extrusion of an airfoil in the spanwise direction) it is always oriented in the spanwise direction, but while in aerodynamics and thin airfoil theory the second axis generating the surface is commonly the chordwise direction:

${\displaystyle S_{aer}\equiv c\,s}$

resulting in a coefficient:

${\displaystyle C_{\mathrm {L} ,\,aer}\equiv {\frac {L}{q\,c\,s}},}$

while for thick airfoils and in marine dynamics, the second axis is sometimes taken in the thickness direction:

${\displaystyle S_{mar}=t\,s}$

resulting in a different coefficient:

${\displaystyle C_{\mathrm {L} ,\,mar}\equiv {\frac {L}{q\,t\,s}}}$

The ratio between these two coefficients is the thickness ratio:

${\displaystyle C_{\mathrm {L} ,\,mar}\equiv {\frac {c}{t}}C_{\mathrm {L} ,\,aer}}$

The lift coefficient can be approximated using the lifting-line theory,[4] numerically calculated or measured in a wind tunnel test of a complete aircraft configuration.

A typical curve showing section lift coefficient versus angle of attack for a cambered airfoil

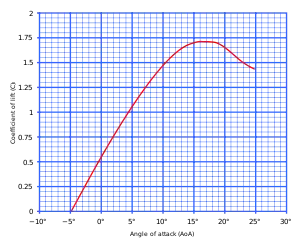
Lift coefficient may also be used as a characteristic of a particular shape (or cross-section) of an airfoil. In this application it is called the section lift coefficient ${\displaystyle c_{\text{l}}}$. It is common to show, for a particular airfoil section, the relationship between section lift coefficient and angle of attack.[5] It is also useful to show the relationship between section lift coefficient and drag coefficient.

The section lift coefficient is based on two-dimensional flow over a wing of infinite span and non-varying cross-section so the lift is independent of spanwise effects and is defined in terms of ${\displaystyle l}$, the lift force per unit span of the wing. The definition becomes

${\displaystyle c_{\text{l}}={\frac {l}{q\,L}},}$

where L is the reference length that should always be specified: in aerodynamics and airfoil theory usually the airfoil chord ${\displaystyle c\,}$ is chosen, while in marine dynamics and for struts usually the thickness ${\displaystyle t\,}$ is chosen. Note this is directly analogous to the drag coefficient since the chord can be interpreted as the "area per unit span".

For a given angle of attack, c_l can be calculated approximately using the thin airfoil theory,[6] calculated numerically or determined from wind tunnel tests on a finite-length test piece, with end-plates designed to ameliorate the three-dimensional effects. Plots of c_l versus angle of attack show the same general shape for all airfoils, but the particular numbers will vary. They show an almost linear increase in lift coefficient with increasing angle of attack with a gradient known as the lift slope. For a thin airfoil of any shape the lift slope is π²/90 ≃ 0.11 per degree. At higher angles a maximum point is reached, after which the lift coefficient reduces. The angle at which maximum lift coefficient occurs is the stall angle of the airfoil, which is approximately 10 to 15 degrees on a typical airfoil.

The stall angle for a given profile is also increasing with increasing values of the Reynolds number, at higher speeds indeed the flow tends to stay attached to the profile for longer delaying the stall condition.[7][8] For this reason sometimes wind tunnel testing performed at lower Reynolds numbers than the simulated real life condition can sometimes give conservative feedback overestimating the profiles stall.

Symmetric airfoils necessarily have plots of c_l versus angle of attack symmetric about the c_l axis, but for any airfoil with positive camber, i.e. asymmetrical, convex from above, there is still a small but positive lift coefficient with angles of attack less than zero. That is, the angle at which c_l = 0 is negative. On such airfoils at zero angle of attack the pressures on the upper surface are lower than on the lower surface.

• Lift-to-drag ratio
• Drag coefficient
• Foil (fluid mechanics)
• Pitching moment
• Circulation control wing
• Zero lift axis

• L. J. Clancy (1975): Aerodynamics. Pitman Publishing Limited, London, ISBN 0-273-01120-0
• Abbott, Ira H., and Doenhoff, Albert E. von (1959): Theory of Wing Sections, Dover Publications New York, # 486-60586-8