Mollweide's formula
In trigonometry, Mollweide's formula is a pair of relationships between sides and angles in a triangle.[1][2]
| Trigonometry |
|---|
 |
| Reference |
| Laws and theorems |
| Calculus |
A variant in more geometrical style was first published by Isaac Newton in 1707 and then by Friedrich Wilhelm von Oppel in 1746. Thomas Simpson published the now-standard expression in 1748. Karl Mollweide republished the same result in 1808 without citing those predecessors.[3]
It can be used to check the consistency of solutions of triangles.[4]
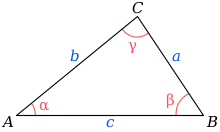
Let a, b, and c be the lengths of the three sides of a triangle. Let α, β, and γ be the measures of the angles opposite those three sides respectively. Mollweide's formulas are
Relation to other trigonometric identities
Because in a planar triangle these identities can alternately be written in a form in which they are more clearly a limiting case of Napier's analogies for spherical triangles,
Dividing one by the other to eliminate results in the law of tangents,
In terms of half-angle tangents alone, Mollweide's formula can be written as
or equivalently
Multiplying the respective sides of these identities gives one half-angle tangent in terms of the three sides,
which becomes the law of cotangents after taking the square root,
where is the semiperimeter.
The identities can also be proven equivalent to the law of sines and law of cosines.
Dual relations
In spherical trigonometry, the law of cosines and derived identities such as Napier's analogies have precise duals swapping central angles measuring the sides and dihedral angles at the vertices. In the infinitesimal limit, the law of cosines for sides reduces to the planar law of cosines and two of Napier's analogies reduce to Mollweide's formulas above. But the law of cosines for angles degenerates to By dividing squared side length by the spherical excess we obtain a non-vanishing ratio, the spherical trigonometry relation:
In the infinitesimal limit, as the half-angle tangents of spherical sides reduce to lengths of planar sides, the half-angle tangent of spherical excess reduces to twice the area of a planar triangle, so on the plane this is:
and likewise for and
As corollaries (multiplying or dividing the above formula in terms of and ) we obtain two dual statements to Mollweide's formulas. The first expresses the area in terms of two sides and the included angle, and the other is the law of sines:
We can alternately express the second formula in a form closer to one of Mollweide's formulas (again the law of tangents):
References
- Ernest Julius Wilczynski, Plane Trigonometry and Applications, Allyn and Bacon, 1914, page 102
- Michael Sullivan, Trigonometry, Dellen Publishing Company, 1988, page 243.
- H. C. Bradley, T. Yamanouti, W. V. Lovitt and R. C. Archibald (1921) "Discussions: Geometric Proofs of the Law of Tangents" The American Mathematical Monthly Vol. 28, No. 11/12, pp. 440-443
- Ernest Julius Wilczynski, Plane Trigonometry and Applications, Allyn and Bacon, 1914, page 105
Further reading
- H. Arthur De Kleine, "Proof Without Words: Mollweide's Equation", Mathematics Magazine, volume 61, number 5, page 281, December, 1988.