Neutron–proton ratio

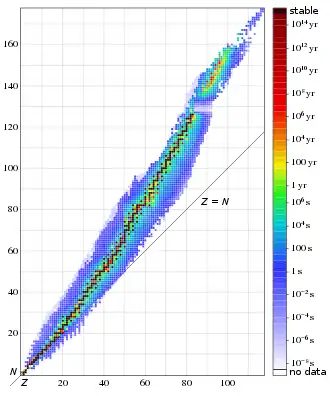
The neutron–proton ratio (N/Z ratio or nuclear ratio) of an atomic nucleus is the ratio of its number of neutrons to its number of protons. Among stable nuclei and naturally occurring nuclei, this ratio generally increases with increasing atomic number.[1] This is because electrical repulsive forces between protons scale with distance differently than strong nuclear force attractions. In particular, most pairs of protons in large nuclei are not far enough apart, such that electrical repulsion dominates over the strong nuclear force, and thus proton density in stable larger nuclei must be lower than in stable smaller nuclei where more pairs of protons have appreciable short-range nuclear force attractions.

Nuclear physics
Models of the nucleus
Nuclides' classification
Nuclear stability
Radioactive decay
Nuclear fission
Capturing processes
High-energy processes
Nucleosynthesis and
nuclear astrophysics
High-energy nuclear physics
Scientists

For each element with atomic number Z small enough to occupy only the first three nuclear shells, that is up to that of calcium (Z = 20), there exists a stable isotope with N/Z ratio of one, with the exception of beryllium (N/Z = 1.25) and every element with odd atomic number between 9 and 19 inclusive (N = Z + 1). Hydrogen-1 (N/Z ratio = 0) and helium-3 (N/Z ratio = 0.5) are the only stable isotopes with neutron–proton ratio under one. Uranium-238 has the highest N/Z ratio of any primordial nuclide at 1.587,[2] while mercury-204 has the highest N/Z ratio of any known stable isotope at 1.55. Radioactive decay generally proceeds so as to change the N/Z ratio to increase stability. If the N/Z ratio is greater than 1, alpha decay increases the N/Z ratio, and hence provides a common pathway towards stability for decays involving large nuclei with too few neutrons. Positron emission and electron capture also increase the ratio, while beta decay decreases the ratio.

Nuclear waste exists mainly because nuclear fuel has a higher stable N/Z ratio than its fission products.

Semi-empirical description

For stable nuclei, the neutron-proton ratio is such that the binding energy is at a local minimum or close to a minimum.

From the liquid drop model, this bonding energy is approximated by empirical Bethe–Weizsäcker formula

Given a value of and ignoring the contributions of nucleon spin pairing (i.e. ignoring the term), the binding energy is a quadratic expression in that is minimized when the neutron-proton ratio is .

Isotope half-lives. Note that the plot for stable isotopes diverges from the line Z = N as the element number Z becomes larger

See also

References
  1. "21.2: Patterns of Nuclear Stability". Chemistry LibreTexts. 2014-11-18. Retrieved 2019-04-10.
  2. "Radioactive Decay". chemed.chem.purdue.edu. Retrieved 2019-04-09.



This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.