Pseudo-deltoidal icositetrahedron
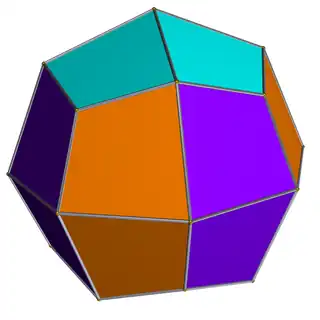
The pseudo-deltoidal icositetrahedron is a convex polyhedron with 24 congruent kites as its faces. It is the dual of the elongated square gyrobicupola, also known as the pseudorhombicuboctahedron.
| Pseudo-deltoidal icositetrahedron | |
|---|---|
.png.webp) (see 3D model) | |
| Type | Johnson dual, pseudo-uniform dual |
| Faces | 24, congruent |
| Face polygon |  Kite with: 1 obtuse angle 3 equal acute angles |
| Edges | 24 short + 24 long = 48 |
| Vertices | 8 of degree 3 18 of degree 4 26 in all |
| Vertex configurations | 4.4.4 (for 8 vertices) 4.4.4.4 (for 2+8+8 vertices) |
| Symmetry group | D4d = D4v, [2+,24], (2*4), order 4×4 |
| Rotation group | D4, [2,4]+, (224), order 2×4 |
| Dihedral angle | same value for short & long edges: |
| Properties | convex, regular vertices[1] |
| Net | .png.webp) (click to enlarge) |
| Dual polyhedron |  |
As the pseudorhombicuboctahedron is tightly related to the rhombicuboctahedron, but has a twist along an equatorial belt of faces (and edges), the pseudo-deltoidal icositetrahedron is tightly related to the deltoidal icositetrahedron, but has a twist along an equator of (vertices and) edges.
Properties
Vertices
As the faces of the pseudorhombicuboctahedron are regular, the vertices of the pseudo-deltoidal icositetrahedron are regular.[1] But due to the twist, these 26 vertices are of four different kinds:
- eight vertices connecting three short edges (yellow vertices in 1st figure below),
- two apices connecting four long edges (top and bottom vertices, light red in 1st figure below),
- eight vertices connecting four alternate edges: short-long-short-long (dark red vertices in 1st figure below),
- eight vertices connecting one short and three long edges (twisted-equator vertices, medium red in 1st figure below).
Edges
A pseudo-deltoidal icositetrahedron has 48 edges: 24 short and 24 long, in the ratio of — their lengths are and respectively, if its dual pseudo-rhombicuboctahedron has unit edge length.[2]
Faces
As the pseudorhombicuboctahedron has only one type of vertex figure, the pseudo-deltoidal icositetrahedron has only one shape of face (it is monohedral); its faces are congruent kites. But due to the twist, the pseudorhombicuboctahedron is not vertex-transitive, with its vertices in two different symmetry orbits (*), and the pseudo-deltoidal icositetrahedron is not face-transitive, with its faces in two different symmetry orbits (*) (it is 2-isohedral); these 24 faces are of two different kinds:
- eight faces with light red, dark red, yellow, dark red vertices (top and bottom faces, light red in 1st figure below),
- sixteen faces with yellow, dark red, medium red, medium red vertices (side faces, blue in 1st figure below).
(*) (three different symmetry orbits if we only consider rotational symmetries)
|
| ||||||||||||||||

References
- "duality". www.polyhedra-world.nc. Retrieved 2022-10-26.
- http://mathworld.wolfram.com/DeltoidalIcositetrahedron.html