Shrikhande graph
In the mathematical field of graph theory, the Shrikhande graph is a graph discovered by S. S. Shrikhande in 1959.[1][2] It is a strongly regular graph with 16 vertices and 48 edges, with each vertex having degree 6. Every pair of nodes has exactly two other neighbors in common, whether the pair of nodes is connected or not.
| Shrikhande graph | |
|---|---|
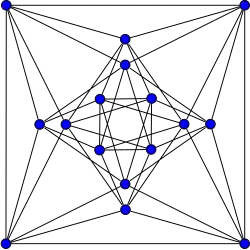 The Shrikhande graph | |
| Named after | S. S. Shrikhande |
| Vertices | 16 |
| Edges | 48 |
| Radius | 2 |
| Diameter | 2 |
| Girth | 3 |
| Automorphisms | 192 |
| Chromatic number | 4 |
| Chromatic index | 6 |
| Book thickness | 4 |
| Queue number | 3 |
| Properties | Strongly regular Hamiltonian Symmetric Eulerian Integral |
| Table of graphs and parameters | |
Construction
The Shrikhande graph can be constructed as a Cayley graph. The vertex set is . Two vertices are adjacent if and only if the difference is in .
Properties
In the Shrikhande graph, any two vertices I and J have two distinct neighbors in common (excluding the two vertices I and J themselves), which holds true whether or not I is adjacent to J. In other words, it is strongly regular and its parameters are: {16,6,2,2}, i.e., . This equality implies that the graph is associated with a symmetric BIBD. The Shrikhande graph shares these parameters with exactly one other graph, the 4×4 rook's graph, i.e., the line graph L(K4,4) of the complete bipartite graph K4,4. The latter graph is the only line graph L(Kn,n) for which the strong regularity parameters do not determine that graph uniquely but are shared with a different graph, namely the Shrikhande graph (which is not a rook's graph).[2][3]
The Shrikhande graph is locally hexagonal; that is, the neighbors of each vertex form a cycle of six vertices. As with any locally cyclic graph, the Shrikhande graph is the 1-skeleton of a Whitney triangulation of some surface; in the case of the Shrikhande graph, this surface is a torus in which each vertex is surrounded by six triangles.[4] Thus, the Shrikhande graph is a toroidal graph. The embedding forms a regular map in the torus, with 32 triangular faces. The skeleton of the dual of this map (as embedded in the torus) is the Dyck graph, a cubic symmetric graph.
The Shrikhande graph is not a distance-transitive graph. It is the smallest distance-regular graph that is not distance-transitive.[5]
The automorphism group of the Shrikhande graph is of order 192. It acts transitively on the vertices, on the edges and on the arcs of the graph. Therefore, the Shrikhande graph is a symmetric graph.
The characteristic polynomial of the Shrikhande graph is : . Therefore, the Shrikhande graph is an integral graph: its spectrum consists entirely of integers.
It has book thickness 4 and queue number 3.[6]
Gallery
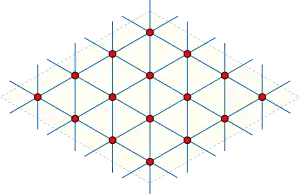 The Shrikhande graph is a toroidal graph.
The Shrikhande graph is a toroidal graph. The chromatic number of the Shrikhande graph is 4.
The chromatic number of the Shrikhande graph is 4. The chromatic index of the Shrikhande graph is 6.
The chromatic index of the Shrikhande graph is 6. The Shrikhande graph drawn symmetrically.
The Shrikhande graph drawn symmetrically. The Shrikhande graph is Hamiltonian.
The Shrikhande graph is Hamiltonian.
Notes
- Weisstein, Eric W. "Shrikhande Graph". MathWorld.
- Shrikhande, S. S. (1959), "The uniqueness of the L2 association scheme", Annals of Mathematical Statistics, 30: 781–798, doi:10.1214/aoms/1177706207, JSTOR 2237417.
- Harary, F. (1972), "Theorem 8.7", Graph Theory (PDF), Massachusetts: Addison-Wesley, p. 79, archived from the original (PDF) on November 9, 2013.
- Brouwer, A. E. Shrikhande graph.
- Brouwer, A. E.; Cohen, A. M.; Neumaier, A. (1989), Distance-Regular Graphs, New York: Springer-Verlag, pp. 104–105 and 136.
- Jessica Wolz, Engineering Linear Layouts with SAT. Master Thesis, University of Tübingen, 2018
References
- Holton, D. A.; Sheehan, J. (1993), The Petersen Graph, Cambridge University Press, p. 270, ISBN 0-521-43594-3.
External links
- The Shrikhande Graph, Peter Cameron, August 2010.