Tonal system

The tonal system is a base 16 system of notation (predating the widespread use of hexadecimal in computing), arithmetic, and metrology proposed in 1859 by John W. Nystrom.[1] In addition to new weights and measures, his proposal included a new calendar with sixteen months, a new system of coinage, and a clock with sixteen major divisions of the day (called tims). Nystrom advocated his system thus:

I am not afraid, or do not hesitate, to advocate a binary system of arithmetic and metrology. I know I have nature on my side; if I do not succeed to impress upon you its utility and great importance to mankind, it will reflect that much less credit upon our generation, upon scientific men and philosophers.[2]

This article is about Nystrom's proposed base 16 notation. For the musical system, see tonality.
Proposed clock-face with noon on top
The proposed figures on the right are based on rotations of those on the left (assigning value 10 to symbol 9).

Names for the numbers

He proposed names for the digits, calling zero "noll" and counting (from one to sixteen):

"An,  de,  ti,  go,  su,  by,  ra,  me,  ni,  ko,  hu,  vy,  la,  po,  fy,  ton." (Therefore, tonal system.)

Because hexadecimal requires sixteen digits, Nystrom supplemented the existing decimal digits 0 through 9 with his own invented characters (shown on his clockface above) and changed the value of 9 to ten. Later, the hexadecimal notation overcame this same obstacle by using the digits 0 through 9 followed by the letters A through F.

The numbers 1116 and 1216 would be said "tonan", "tonde", etc. The table below shows Nystrom's names for successive powers of 1016.

Base 16 Number Tonal Name Base 10 Equivalent
10 ton 16
100 san 256
1000 mill 4,096
1,0000 bong 65,536
10,0000 tonbong 1,048,576
100,0000 sanbong 16,777,216
1000,0000 millbong 268,435,456
1,0000,0000 tam 4,294,967,296
1,0000,0000,0000 song 16^12
1,0000,0000,0000,0000 tran 16^16
1,0000,0000,0000,0000,0000 bongtran 16^20

Thus, the hexadecimal number 1510,0000 would be "mill-susanton-bong". This first hexadecimal system, proposed in the 19th century, has thus far not achieved widespread usage.

Although Nystrom did not propose a language for tonal fractions, his nomenclature for units of measure does provide one: the name of a power of sixteen before the base unit's name multiplies it by that number, but a power of sixteen after the base unit's name divides it by that number. Thus, de timtons means 1⁄8 tim.

Geography
Nystrom's hexadecimal compass

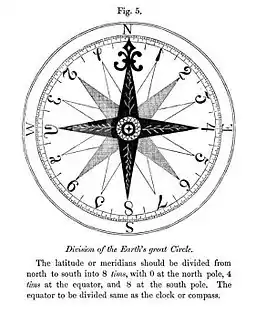
For latitudes he put 0 at the North Pole, 4 at the equator and 8 at the South Pole. The units were called tims. They are the same as the colatitudes measured in turns times 16.

Tonal (in tims) ISO 6709 Colatitude (in degrees) Colatitude (in turns)
0 090 0° 0
1 67.5
2 045 45° 0.125
3 022.5
4 000 90° 0.25
5 −22.5
6 −045 135° 0.375
7 −67.5
8 −090 180° 0.5

Music
A page in the tonal system book

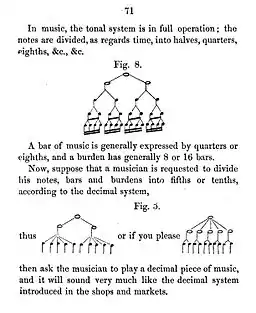
In his book he made a reference to music notation, where binary division is already in use for time. He also discussed the problem of pitch inflation, which he proposed to solve by setting the A below middle C to a frequency of san per timmill (194 Hz).

References
  1. Nystrom, John W. Project of a New System of Arithmetic, Weight, Measure and Coins, Proposed to be Called the Tonal System, with Sixteen to the Base
  2. (Quotation: John W. Nystrom, ca. 1863) The Art of Computer Programming section 4.1, Donald Knuth.
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.