Electron diffraction

In order to be diffracted, electrons need to interact with matter. Due to their negative electric charge, their interactions differ from other radiation used in diffraction studies of materials such as X-rays and neutrons. Negative electrons are scattered due to Coulomb forces when they interact with a positively charged atomic core. In comparison, X-rays are scattered after interactions with valence electrons, while neutrons are scattered by the atomic nuclei through the strong nuclear force.[13]

Electron diffraction occurs as the result of an elastic scattering, when the incident electrons do not lose their kinetic energy in their interactions with atoms. In some cases, however, even inelastically scattered electrons can be diffracted as the result of a following elastic interaction.

Due to the wave-particle duality, electrons behave as particles as well as waves. A basic characteristic of the wave is a wavelength. The electron wavelength is given by de Broglie equation as[10]

$${\displaystyle \lambda ={\frac {h}{p}}.}$$

Here ${\displaystyle h}$ is Planck's constant and ${\displaystyle p=mv}$[14] is momentum, where ${\displaystyle m}$ is the electron mass and ${\displaystyle v}$ the velocity. Since the electrons are charged particles, they can be accelerated using electric potential. This allows the electrons to get accelerated to speeds, where relativistic theory needs to be applied. Therefore, there are two definitions of wavelength - non-relativistic and relativistic.

In a non-relativistic theory, the electrons accelerated in an electric potential ${\displaystyle V}$ gain the velocity ${\displaystyle v}$ given by the equation[14]

$${\displaystyle {\frac {v}{c}}={\sqrt {\frac {2eV}{m_{0}c^{2}}}},}$$

where ${\displaystyle m_{0}}$ is the electron rest mass, ${\displaystyle e}$ is the elementary charge and ${\displaystyle c}$ is the speed of light. Substituting the momentum and velocity to the de Broglie equation we receive

$${\displaystyle \lambda ={\frac {h}{p}}={\frac {h}{m_{0}v}}={\frac {h}{\sqrt {2m_{0}eV}}}.}$$

Relativistic wavelengths for various accelerating voltages[15]

${\displaystyle V}$ [kV]	${\displaystyle \lambda }$ [pm]
0.1	122.633
0.5	54.832
1	38.763
10	12.204
20	8.588
50	5.355
100	3.701
200	2.508
300	1.969
400	1.644
800	1.027
1000	0.872
2000	0.504
3000	0.357

In an electron microscope, the accelerating potential is usually several thousand volts, causing the electron to travel at an appreciable fraction of the speed of light. Scanning electron microscopes typically operate at an accelerating voltage of 10,000 volts (10 kV) giving an electron velocity approximately 20% of the speed of light, while a typical TEM operates at 200 kV raising the electron velocity to 70% the speed of light. Therefore, relativistic effects need to be taken into account. The relativistic relation between energy and momentum is[14]

${\displaystyle E_{T}^{2}=(m_{0}c^{2}+eV)^{2}=p^{2}c^{2}+m_{0}^{2}c^{4}.}$

It follows then, that the ratio between the electron mass and its rest mass (or Lorentz factor) is[14]

${\displaystyle {\frac {m}{m_{0}}}=\gamma ={\frac {1}{\sqrt {1-{\frac {v^{2}}{c^{2}}}}}}=1+{\frac {eV}{m_{0}c^{2}}}.}$

Then the relativistic velocity is given by the equation[14]

${\displaystyle {\frac {v}{c}}={\frac {[eV(eV+2m_{0}c^{2})]^{1/2}}{m_{0}c^{2}+eV}}.}$

Substitution of the De Broglie equation to the above expression of energy ${\displaystyle E_{T}}$ gives[14]

${\displaystyle (m_{0}c^{2}+eV)^{2}=({\frac {hc}{\lambda }})^{2}+m_{0}^{2}c^{4},}$

which leads to the final expression for the relativistic wavelength[14]

${\displaystyle \lambda ={\frac {hc}{\sqrt {eV(2m_{0}c^{2}+eV)}}}.}$

The wavelength of the electrons in a 10 kV SEM is then 12.2 × 10⁻¹² m (12.2 pm) while in a 200 kV TEM the wavelength is 2.5 pm. In comparison, the wavelength of X-rays usually used in X-ray diffraction is in the order of 100 pm (Cu Kα: λ=154 pm).

Wavelength of the electron beam used in a typical electron microscope is sufficiently small, that crystal lattice acts as a diffraction grating. Therefore a diffraction pattern can be formed with beams diffracted under certain angles and intensities.

Electron beam incident normally to atomic structure is transmitted (${\displaystyle n}$=1) and diffracted under specific angles (${\displaystyle n}$ equal to 1 and 2).

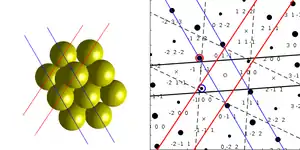
Due to the diffraction, part of the electrons is scattered at particular angles (diffracted beams), while others pass through the sample without changing their direction (transmitted beams). In order to determine the diffraction angles, the electron beam normally incident to the atomic lattice can be seen as a planar wave, which is re-transmitted by each atom as a spherical wave. Due to the constructive interference, the spherical waves from number of diffracted beams under angles ${\displaystyle \theta _{n}}$ given by the equation

${\displaystyle d\sin \theta _{n}=n\lambda ,}$

where the integer ${\displaystyle n}$ is an order of diffraction and ${\displaystyle d}$ is the distance between atoms if we only assume one row of atoms as shown in the illustration aside. For a real-world 3D atomic structure, it would be an interplanar distance ${\displaystyle d_{hkl}}$ of plane with Miller indices ${\displaystyle (hkl)}$, which needs to be oriented parallel to the electron beam.

In the kinematic theory of diffraction, the intensity of a diffracted beam corresponding to a plane with Miller indices ${\displaystyle (hkl)}$ is proportional to sqare of the structure factor, which is defined as

${\displaystyle F_{hkl}=\sum _{j=1}^{N}f_{j}\mathrm {e} ^{[2\pi i(hx_{j}+ky_{j}+\ell z_{j})]},}$

where the sum is over all atoms in the unit cell, ${\displaystyle x_{j},y_{j},z_{j}}$ are the positional coordinates of the ${\displaystyle j}$-th atom, and ${\displaystyle f_{j}}$ is the atomic scattering factor of the ${\displaystyle j}$-th atom.[16]

Kinematical theory expects the electron to interact with the matter only once. For many applications, this does not pose significant limitations. For study of more complex diffraction phenomenons, dynamical simulations using for instance Multislice or Bloch waves is needed.[14]

Relation between spot and ring diffraction illustrated on 1 to 1000 grains of MgO using simulation engine of CrysTBox. Corresponding experimental image can be seen below.

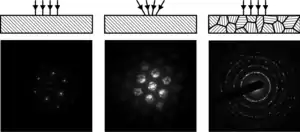
Character of the resulting diffraction pattern depends on whether the beam is diffracted by one single crystal or by number of differently oriented crystallites for instance in a polycrystalline material. The single-crystalline diffractogram depicts a regular pattern of bright spots. This pattern can be seen as a two-dimensional projection of reciprocal crystal lattice. If there are more contributing crystallites, the diffraction image becomes a superposition of individual crystals' diffraction patterns. Ultimately, this superposition contains diffraction spots of all possible crystallographic plane systems in all possible orientations. These conditions result in a diffractogram of concentric rings of discrete radii for the following reasons:

1. The discreteness of ring radii is given by the fact, that there are discrete spacings between various parallel crystallographic planes in given crystal system and therefore the beams satisfying the diffraction condition can only form diffraction spots in discrete distances from the transmitted beam.
2. The concentricity and ringlike shape is given by the fact, that there are all possible orientations of crystallographic planes and therefore the diffraction spots are formed all around the transmitted beam (rings centre) at the distance (ring radius) corresponding to particular crystalographic plane.

Crystallographic planes in [0 1 1] Mg cell with corresponding diffraction spots and Kikuchi lines simulated in CrysTBox

Kikuchi lines are linear diffractogram features created by electrons scattered first inelastically and then elastically. As the electron beam interacts with matter, some electrons are diffracted via elastic scattering, while other can be scattered inelastically losing part of their kinetic energy. Direction of inelastically scattered electrons is significantly less predictive - they can more or less follow the direction of the original beam as well as be "reflected" back. In all cases, they continue to interact with the matter and some of them are diffracted. These electrons then form Kikuchi lines.[17]

Kikuchi lines are paired forming Kikuchi bands. They are indexed after the crystallographic planes they were formed with. Angular width of the band is equal to the diffraction angle ${\displaystyle \theta _{1}}$ specified above which means, that in the diffractogram, the width of ${\displaystyle (hkl)}$ plane will be equal to the distance between transmitted beam and ${\displaystyle (hkl)}$ diffraction spot. The position of Kikuchi bands is fixed against each other but not against the diffraction spots. As the crystal is tilted in the electron beam, the bands move on the diffraction pattern.[17]

Diffraction can be also performed on individual molecules dispersed in a gaseous atmosphere. Since the beam is dffracted by multiple molecules with all possible orientations relative to the beam, the resulting scattering pattern consists of concentric rings analogously to the diffraction cones produced by polycrystalline materials described above.

In gas electron diffraction (GED) the diffraction intensities at a particular diffraction angle ${\displaystyle \theta }$ are described via so-called scattering variable defined as

${\textstyle |s|={\frac {4\pi }{\lambda }}\sin \left({\frac {\theta }{2}}\right).}$

The total intensity is then given as a sum of partial contributions:

${\displaystyle I_{\text{tot}}(s)=I_{a}(s)+I_{m}(s)+I_{t}(s)+I_{b}(s),}$

where ${\displaystyle I_{a}(s)}$ results from scattering by individual atoms, ${\displaystyle I_{m}(s)}$ by pairs of atoms and ${\displaystyle I_{t}(s)}$ by atom triplets. Intensity ${\displaystyle I_{b}(s)}$ corresponds to the background which, unlike the previous contributions, must be determined experimentally. Intensity of atomic scattering ${\displaystyle I_{a}(s)}$ is defined as

$${\displaystyle I_{a}(s)={\frac {K^{2}}{R^{2}}}I_{0}\sum _{i=1}^{N}|f_{i}(s)|^{2},}$$

where ${\displaystyle K=(8\pi ^{2}me^{2})/h^{2}}$, ${\displaystyle R}$ is the distance between the scattering object detector, ${\displaystyle I_{0}}$ is the intensity of the primary electron beam and ${\displaystyle f_{i}(s)}$ is the scattering amplitude of the i-th atom in the molecular structure in the experiment. ${\displaystyle I_{a}(s)}$ is the main contribution and easily obtained for known gas composition.

The most valuable information is carried by the intensity of molecular scattering ${\displaystyle I_{a}(s)}$, as it contains information about the distance between all pairs of atoms in the molecule, whether bonded or not. It is given by formula

${\displaystyle I_{m}(s)={\frac {K^{2}}{R^{2}}}I_{0}\sum _{i=1}^{N}\sum _{\stackrel {j=1}{i\neq j}}^{N}\left|f_{i}(s)\right|\left|f_{j}(s)\right|{\frac {\sin[s(r_{ij}-\kappa s^{2})]}{sr_{ij}}}e^{-(1/2l_{ij}s^{2})}\cos[\eta _{i}(s)-\eta _{i}(s)],}$

where ${\displaystyle r_{ij}}$ is the distance between two atoms, ${\displaystyle l_{ij}}$ is the mean square amplitude of vibration between the two atoms, ${\displaystyle \kappa }$ is the anharmonicity constant and ${\displaystyle \eta }$is a phase factor which is important for atomic pairs with very different nuclear charges. The summation is performed over all atom pairs. Atomic triplet intensity ${\displaystyle I_{t}(s)}$ is negligible in most cases.

Diffraction patterns with different crystallinity and beam convergence. From left: spot diffraction, CBED, ring diffraction

Electron diffraction in a Transmission electron microscopy (TEM) is a versatile technique allowing to determine a wide range of crystallographic quantities. The versatility of TEM originates from its ability to form the electron beam using complex electron optics. Good control of the beam geometry allows to perform various diffraction techniques enabling to measure the crystal lattice constants, study crystal defects or even to reconstruct an unknown crystal structure.

Even though the diffraction analysis in TEM is a strong analytical tool itself, it can be supported by other tools available in TEM either naturally or as an optional equipment. Among other methods, TEM provides magnified sample image or even high-resolution image, chemical analysis through energy-dispersive X-ray spectroscopy, investigations of electronic structure and bonding through electron energy loss spectroscopy, and studies of the mean inner potential through electron holography.

Compared to another widely used material characterization technique, X-ray diffraction, TEM analysis is significantly more localized. Due to the longer radius of Ewald sphere, electron diffraction also offers constructive interference in a broader diffractogram area resulting in bright diffraction spots in higher distances from the optical axis.

Imaging scheme of magnetic lens (center) with magnified image (left) and diffraction pattern (right) formed in back focal plane

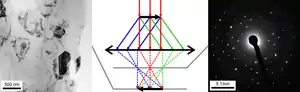
In TEM, electron beam passes through a thin film of the examined material. Before and after its interaction with the sample, the beam is formed by various elements of electron optics including magnetic lenses, deflectors or apertures. Optical elements above the sample are used to control the incident beam geometry allowing for a wide and parallel beam, focused nanoscopic probe or convergent cone-shaped beam. As it interacts with the sample, part of the beam is diffracted and part is transmitted through the sample without changing its direction.

Below the sample, the beam is deflected by the magnetic lens. Each set of initially parallel rays intersect at certain point in the back focal plane forming a diffraction pattern. The transmitted rays intersect right in the optical axis. The diffracted rays intersect at certain distance from the optical axis (corresponding to interplanar distance of the planes diffracting the beam) and under certain azimuth (corresponding to the orientation of the planes diffracting the beam). In case or parallel incident beam, those intersections can be seen as bright diffraction spots typical for selected area diffraction. In the image plane below the back focal plane, a magnified image of the sample is formed. Further electron optics allows to select which plane is projected to the microscope camera and, therefore, whether a magnified image or diffractogram is acquired. Modern microscopes allow to switch between the imaging and diffraction mode by pressing a single button, which makes diffraction data easily available and accessible.[13]

Single-crystalline SADP automatically interpreted with CrysTBox software.

The simplest diffraction technique in TEM is selected area (electron) diffraction (SAED) for which the incident beam is wide and parallel. In order to select particular region of interest from which the diffraction should be received, a selected area aperture is used. It is located right below the sample and can be positioned so that it only allows to pass the wanted portion of the beam blocking the rest. This way, the analysis can be limited for instance to individual crystallites.

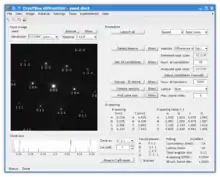
If a parallel beam is used to acquire a diffraction pattern from a single-crystal, the resulting image can be seen as a two-dimensional projection of the crystal reciprocal lattice. The depicted diffraction spots correspond to individual crystallographic planes which satisfy diffraction conditions. This allows to determine interplanar distances and angles or crystal symmetry. In combination with modern automated analytical software such as CrysTBox, SAED can be used for a quantitative analysis with picometric precision.[18]

Diffraction pattern of magnesium simulated using CrysTBox for various crystal orientations.

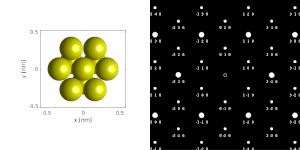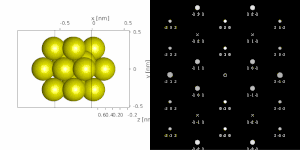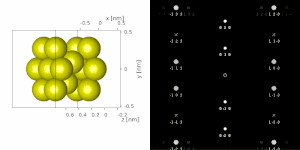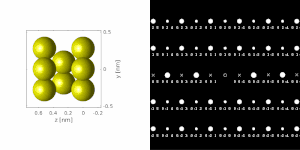
If the sample is tilted against the electron beam, diffraction conditions are satisfied for different set of crystallographic planes yielding different constellation of diffraction spots. This allows to determine the crystal orientation, which can be used for instance to set the orientation needed for particular experiment, to determine misorientation between adjacent grains or crystal twins.[15][18] Since different sample orientations result in different projections of the reciprocal lattice, they provide an opportunity to reconstruct the three-dimensional information about the crystal structure lost in individual projections. A series of diffractograms varying in tilt can be acquired and processed using a diffraction tomography analysis in order to reconstruct an unknown crystal structure.[19]

Kikuchi lines occur in TEM especially in thicker samples, where inelastic scattering followed by the elastic one occurs with sufficient frequency. Since the position of Kikuchi bands within the diffractogram is quite sensitive to crystal orientation, they can be used to fine-tune a zone-axis orientation. Alternatively, they allow to determine crystal orientation with significantly higher accuracy than what is feasible with a spot diffraction analysis. Since mutual positions and orientations of Kikuchi bands is fixed and since the bands intersect in low-index zone axes, they can be used for navigation when changing the orientation between zone axes connected by some band. For those purposes, Kikuchi maps are available.[13]

Ring diffraction image of MgO as recorded (left) and processed with CrysTBox ringGUI (right). Corresponding simulated pattern can be seen above.

If the illuminated area selected by the aperture covers many differently oriented crystallites, their diffraction patterns superimpose forming an image of concentric rings. The ring diffractogram is typical for polycrystalline samples, powders or nanoparticles. A diameter of each ring corresponds to interplanar distance of a plane system present in the sample. Instead of information about individual grains or sample orientation, this diffractogram provides more of a statistical information for instance about overall crystallinity or texture. Textured materials can be recognized by a non-uniform distribution of intensity along the ring circumference despite sufficient crystallinity. Ring diffractograms can be also used to discriminate between nanocrystalline and amorphous phases.[15]

Apart from limiting the beam using the selected area aperture, the site-selectivity can be achieved by condensing the incident beam into a narrow electron probe. This technique is called microprobe or nanoprobe diffraction or simply nanodiffraction. Probes of diameter smaller than 1 nm can be obtained.[13] At these extremes the probe dimension comes at the price of the beam becoming nearly parallel, even though microprobe can be achieved with parallel beam.[15] Compared to SAED, the resulting diffraction spots can be significantly broader and blurry, making a manual analysis quite inaccurate. Accurate processing is possible with automated software like CrysTBox, which is able to take into account a number of diffraction reflections across the whole pattern significantly improving accuracy and repeatability of the analysis.[18]

In Convergent Beam Electron Diffraction (CBED), the incident electrons are focused in a converging cone-shaped beam with the crossover located in the sample volume. Unlike the parallel beam, the convergent beam is able to carry information from the sample volume, not just a two-dimensional projection available in SAED. With convergent beam there is also no need for the selected area aperture, as it is inherently site-selective since the beam crossover is positioned to the object plane where the sample is located.[17]

A CBED pattern consists of disks arranged exactly the same as the spots in SAED. Intensity within the disks, however, is not uniform, but depicts various features and symmetries reflecting the sample structure. Even though the zone axis and lattice parameter analysis based on disk positions does not significantly differ from SAED, the analysis of disks content is significantly more complex. Due to a number of contributing factors, simulation based on dynamical diffraction theory is often required. With appropriate analysis, however, CBED patterns can be used for indexation of the crystal point group, space group identification, measurement of lattice parameters, thickness or strain.[17]

The disk diameter can be controlled using the beam convergence angle. The larger is the angle, the broader the disks are depicting more features. If the angle is increased to certain level, the disks begin to overlap deteriorating the contained information. This can be solved for instance via a large angle convergent electron beam diffraction (LACBED) where the sample is moved upwards or downwards. There are applications, however, where the overlapping disks are beneficial. Ronchigram could serve as an example. It is a CBED pattern of an amorphous sample depicting many intentionally overlapping disks blended into one image providing information about electron optical system.[13]

GED pattern of benzene.

Gas electron diffraction (GED) can be used to determine geometry of molecules dispersed in gases. A gas carrying the examined molecules is exposed to the electron beam, which is diffracted on the molecules. Since the diffracting molecules are randomly oriented, the resulting diffraction pattern consists of concentric rings. The experimentally acquired diffraction intensity is a summation of several components such as background, atomic intensity or molecular intensity.

The most valuable information about the molecular geometry is carried by the molecular intensity ${\displaystyle I_{m}(s)}$, which is specific to pairs of molecular atoms as described above in Theory. If this intensity is derived from an experimental diffractogram by subtracting other contributing intensities, it can be used to match and refine a structural model against the experimental data using an appropriate software.

As an alternative to GED, microwave spectroscopy can be used.

EBSD pattern of silicone.

In Scanning electron microscope the sample surface is mapped using a scanning electron beam. The diffraction image is formed using an electron backscatter diffraction (EBSD). A thin layer of the sample material is penetrated by the electrons, some of which are reflected due to the interactions inside the material and are diffracted on their journey out of the sample. As result of the inelastic scattering followed by the elastic one, typical features for an EBSD image are Kikuchi lines. Since the position of Kikuchi bands is highly sensitive to the crystal orientation, EBSD data acquired using the scanning beam can be used to determine the crystal orientation at particular location on the sample surface. The data are processed by an automated software allowing to routinely generate two-dimensional orientation maps across the sample surface. As the Kikuchi lines carry information about the interplanar angles and distances and, therefore, about the crystal structure, they can be also used for a phase identification or strain analysis.[20]