Sometimes it is helpful to think of complex numbers in a different geometric way. The previous geometric idea where the number
In polar coordinates, the parameters are
The other parameter is the angle
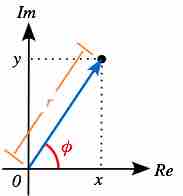
The parameters for polar coordinates
The angle
Multiplying Complex Numbers in Polar Coordinates
So if
For example, consider the complex numbers
So
Then
When we multiply
Perhaps more easily we could multiply
Realizing that we are getting the number whose distance from the origin is
Dividing Complex Numbers in Polar Coordinates
Similarly, if
In other words, when dividing by a complex number, the result is a number whose distance from the origin is the quotient of the distances of the two numbers from the origin, and whose angle with the horizontal is the difference of the angles with the horizontal of the two numbers.
For example, If you were to divide
The result is one unit from the origin and at an angle of
This way of thinking about multiplying and dividing complex numbers gives a geometric way of thinking about those operations.