Derivatives and Rates of Change

Historically, the primary motivation for the study of differentiation was the tangent line problem, which is the task of, for a given curve, finding the slope of the straight line that is tangent to that curve at a given point. The word tangent comes from the Latin word tangens, which means touching. Thus, to solve the tangent line problem, we need to find the slope of a line that is "touching" a given curve at a given point, or, in modern language, that has the same slope. But what exactly do we mean by "slope" for a curve?

The simplest case is when $y$ is a linear function of x, meaning that the graph of $y$ divided by $x$ is a straight line. In this case, $y = f(x) = m x + b$, for real numbers m and b, and the slope m is given by:

$\displaystyle{m = \frac{\Delta y}{\Delta x}}$

where the symbol $\Delta$ (the uppercase form of the Greek letter Delta) means, and is pronounced, "change in." This formula is true because:

$y + \Delta y = f(x+ \Delta x) = m (x + \Delta x) + b = m x + b + m \Delta x = y + m\Delta x$

It follows that $\Delta y = m \Delta x$.

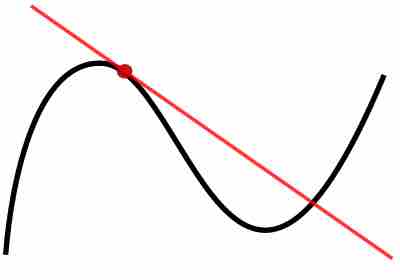
Slope of a function

A function with the slope shown for a given point.

This gives an exact value for the slope of a straight line. If the function $f$ is not linear (i.e., its graph is not a straight line), however, then the change in $y$ divided by the change in $x$ varies: differentiation is a method to find an exact value for this rate of change at any given value of $x$. In other words, differentiation is a method to compute the rate at which a dependent output $y$ changes with respect to the change in the independent input $x$. This rate of change is called the derivative of $y$ with respect to $x$. In more precise language, the dependence of $y$ upon x means that $y$ is a function of $x$. This functional relationship is often denoted $y=f(x)$, where $f$ denotes the function. If $x$ and $y$ are real numbers, and if the graph of $y$ is plotted against $x$, the derivative measures the slope of this graph at each point.