A Microscopic View: Drift Speed

Drift Speed

Electrical signals are known to move very rapidly. Telephone conversations carried by currents in wires cover large distances without noticeable delays. Lights come on as soon as a switch is flicked. Most electrical signals carried by currents travel at speeds on the order of 10⁸m/s, a significant fraction of the speed of light. Interestingly, the individual charges that make up the current move much more slowly on average, typically drifting at speeds on the order of 10⁻⁴m/s.

The high speed of electrical signals results from the fact that the force between charges acts rapidly at a distance. Thus, when a free charge is forced into a wire, the incoming charge pushes other charges ahead of it, which in turn push on charges farther down the line. The resulting electrical shock wave moves through the system at nearly the speed of light. To be precise, this rapidly moving signal or shock wave is a rapidly propagating change in the electric field .

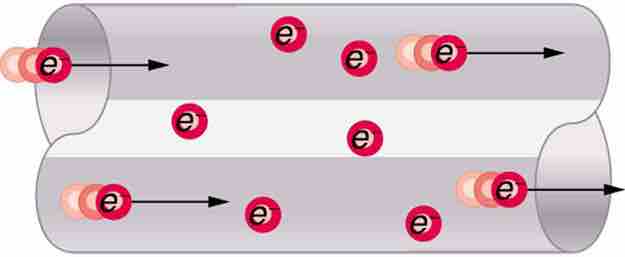
Electrons Moving Through a Conductor

When charged particles are forced into this volume of a conductor, an equal number are quickly forced to leave. The repulsion between like charges makes it difficult to increase the number of charges in a volume. Thus, as one charge enters, another leaves almost immediately, carrying the signal rapidly forward.

Drift Velocity

Good conductors have large numbers of free charges in them. In metals, the free charges are free electrons. The distance that an individual electron can move between collisions with atoms or other electrons is quite small. The electron paths thus appear nearly random, like the motion of atoms in a gas. However, there is an electric field in the conductor that causes the electrons to drift in the direction shown (opposite to the field, since they are negative). The drift velocity v_dis the average velocity of the free charges after applying the field. The drift velocity is quite small, since there are so many free charges. Given an estimate of the density of free electrons in a conductor (the number of electrons per unit volume), it is possible to calculate the drift velocity for a given current. The larger the density, the lower the velocity required for a given current .

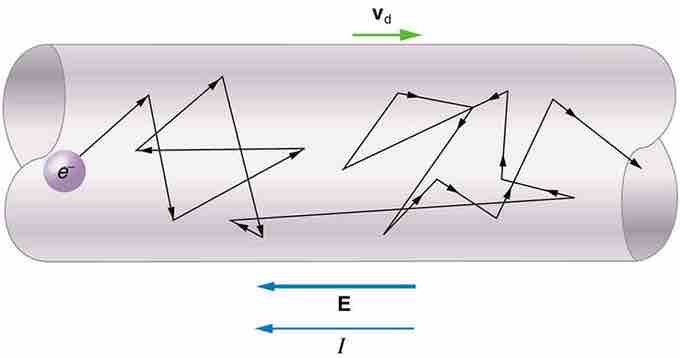
Drift Speed

Free electrons moving in a conductor make many collisions with other electrons and atoms. The path of one electron is shown. The average velocity of the free charges is called the drift velocity and is in the direction opposite to the electric field for electrons. The collisions normally transfer energy to the conductor, requiring a constant supply of energy to maintain a steady current.

It is possible to obtain an expression for the relationship between the current and drift velocity by considering the number of free charges in a segment of wire. The number of free charges per unit volume is given the symbol n and depends on the material. Ax is the volume of a segment, so that the number of free charges in it is nAx. The charge ΔQ in this segment is thus qnAx, where q is the amount of charge on each carrier. (Recall that for electrons, q is 1.60×10−19C. ) The current is the charge moved per unit time. Thus, if all the original charges move out of this segment in time t, the current is:

$I = \Delta Q/\Delta t = qnAx/\Delta t$

Notably, x/Δt is the magnitude of the drift velocity v_d, since the charges move an average distance x in a time t. Rearranging terms gives: I = qnAv_d, where I is the current through a wire of cross-sectional area A made of a material with a free charge density n. The carriers of the current each have charges q and move with a drift velocity of magnitude v_d.

Current density is the electric current per unit area of cross-section. It has units of Amperes per square meter.