Pressure is an important physical quantity—it plays an essential role in topics ranging from thermodynamics to solid and fluid mechanics. As a scalar physical quantity (having magnitude but no direction), pressure is defined as the force per unit area applied perpendicular to the surface to which it is applied. Pressure can be expressed in a number of units depending on the context of use .
Units, Equations and Representations
In SI units, the unit of pressure is the Pascal (Pa), which is equal to a Newton / meter2 (N/m2). Other important units of pressure include the pound per square inch (psi) and the standard atmosphere (atm). The elementary mathematical expression for pressure is given by:
where p is pressure, F is the force acting perpendicular to the surface to which this force is applied, and A is the area of the surface. Any object that possesses weight, whether at rest or not, exerts a pressure upon the surface with which it is in contact. The magnitude of the pressure exerted by an object on a given surface is equal to its weight acting in the direction perpendicular to that surface, divided by the total surface area of contact between the object and the surface. shows the graphical representations and corresponding mathematical expressions for the case in which a force acts perpendicular to the surface of contact, as well as the case in which a force acts at angle θ relative to the surface.

Representation of Pressure
This image shows the graphical representations and corresponding mathematical expressions for the case in which a force acts perpendicular to the surface of contact, as well as the case in which a force acts at angle θ relative to the surface.
Pressure as a Function of Surface Area
Since pressure depends only on the force acting perpendicular to the surface upon which it is applied, only the force component perpendicular to the surface contributes to the pressure exerted by that force on that surface. Pressure can be increased by either increasing the force or by decreasing the area or can oppositely be decreased by either decreasing the force or increasing the area. illustrates this concept. A rectangular block weighing 1000 N is first placed horizontally. It has an area of contact (with the surface upon which it is resting) of 0.1 m2, thus exerting a pressure of 1,000 Pa on that surface. That same block in a different configuration (also in Figure 2), in which the block is placed vertically, has an area of contact with the surface upon which it is resting of 0.01 m2, thus exerting a pressure of 10,000 Pa—10 times larger than the first configuration due to a decrease in the surface area by a factor of 10.
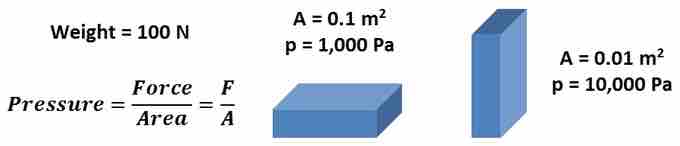
Pressure as a Function of Surface Area
Pressure can be increased by either increasing the force or by decreasing the area or can oppositely be decreased by either decreasing the force or increasing the area.
A good illustration of this is the reason a sharp knife is far more effective for cutting than a blunt knife. The same force applied by a sharp knife with a smaller area of contact will exert a much greater pressure than a blunt knife having a considerably larger area of contact. Similarly, a person standing on one leg on a trampoline causes a greater displacement of the trampoline than that same person standing on the same trampoline using two legs—not because the individual exerts a larger force when standing on one leg, but because the area upon which this force is exerted is decreased, thus increasing the pressure on the trampoline. Alternatively, an object having a weight larger than another object of the same dimensionality and area of contact with a given surface will exert a greater pressure on that surface due to an increase in force. Finally, when considering a given force of constant magnitude acting on a constant area of a given surface, the pressure exerted by that force on that surface will be greater the larger the angle of that force as it acts upon the surface, reaching a maximum when that force acts perpendicular to the surface.
Liquids and Gases: Fluids
Just as a solid exerts a pressure on a surface upon which it is in contact, liquids and gases likewise exert pressures on surfaces and objects upon which they are in contact with. The pressure exerted by an ideal gas on a closed container in which it is confined is best analyzed on a molecular level. Gas molecules in a gas container move in a random manner throughout the volume of the container, exerting a force on the container walls upon collision. Taking the overall average force of all the collisions of the gas molecules confined within the container over a unit time allows for a proper measurement of the effective force of the gas molecules on the container walls. Given that the container acts as a confining surface for this net force, the gas molecules exert a pressure on the container. For such an ideal gas confined within a rigid container, the pressure exerted by the gas molecules can be calculated using the ideal gas law:
where n is the number of gas molecules, R is the ideal gas constant (R = 8.314 J mol-1 K-1), T is the temperature of the gas, and V is the volume of the container.
The pressure exerted by the gas can be increased by: increasing the number of collisions of gas molecules per unit time by increasing the number of gas molecules; increasing the kinetic energy of the gas by increasing the temperature; or decreasing the volume of the container. offers a representation of the ideal gas law, as well as the effect of varying the equation parameters on the gas pressure. Another common type of pressure is that exerted by a static liquid or hydrostatic pressure. Hydrostatic pressure is most easily addressed by treating the liquid as a continuous distribution of matter, and may be considered a measure of energy per unit volume or energy density. We will further discuss hydrostatic pressure in other sections.

Pressure of an Ideal Gas
This image is a representation of the ideal gas law, as well as the effect of varying the equation parameters on the gas pressure.