Adding and subtracting fractions should be a familiar process, and we will rely on this concept in our discussion of adding and subtracting rational expressions.

$\displaystyle \frac 1 2 +\frac 1 3 = \frac 3 6 + \frac 2 6 = \frac 5 6$

The key is finding the least common denominator of the two rational expressions: the smallest multiple of both denominators. Then, you rewrite the two fractions using this denominator. Finally, you add (or subtract) the fractions by combining the numerators and leaving the denominator alone.

But how do you find the least common denominator? 

Consider this problem:

$\displaystyle \frac 5 {12} + \frac 7 {30} = ?$

You could probably find the least common denominator if you played around with the numbers long enough. Here, we will show you a systematic method for finding least common denominators—a method that works with rational expressions just as well as it does with numbers. 

Finding the Least Common Denominator

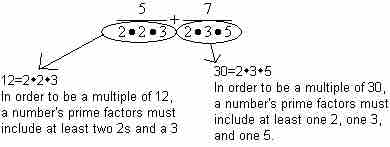
We start, as usual, by factoring. For each of the denominators, we find all the prime factors—i.e., the prime numbers that multiply to give that number.

$\displaystyle \frac 5 {2 \cdot 2 \cdot 3} + \frac 7 {2 \cdot 3 \cdot 5}$

If you are not familiar with the concept of prime factors, it may take a few minutes to get used to. $2\cdot 2 \cdot 3$ is $12$ broken into its prime factors: that is, it is the list of prime numbers that when multiplied together yield 12. Similarly, the prime factors of 30 are 2, 3, and 5. But why does this help? 

Because $12=2 \cdot 2 \cdot 3$, any number whose prime factors include two 2s and one 3 will be a multiple of 12. Similarly, any number whose prime factors include a 2, a 3, and a 5 will be a multiple of 30. See below for further details.

Prime Factors of Fractions

Finding the prime factors of the denominators of two fractions enables us to find a common denominator.

The least common denominator is the smallest number that contains the overlap of both factored denominators: in this case, it must have two 2s, one 3, and one 5. Hence, the least common denominator must be $2 \cdot 2 \cdot 3 \cdot 5 = 60$. 

Now we can finish the problem:

$\begin{aligned} \frac {5} {2 \cdot 2 \cdot 3} + \frac {7} {2 \cdot 3 \cdot 5} &= \left(\frac {5} {2 \cdot 2 \cdot 3} \cdot \frac {5} {5} \right) + \left(\frac {7} {2 \cdot 3 \cdot 5} \cdot \frac {2} {2}\right) \\ &= \frac {25} {60} + \frac {14} {60}\\ &= \frac {39} {60} \\ &= \frac {13} {20} \end{aligned}$

This may look like a very strange way of solving problems that you have known how to solve since the third grade. However, you should spend a few minutes carefully following the above solution. Once you understand why $2 \cdot 2 \cdot 3 \cdot 5 = 60$ is guaranteed to be the least common denominator, you have the key concept required to add and subtract rational expressions.

Addition and Subtraction of Rational Expressions

When applying this strategy to rational expressions, first look at the denominators of the two rational expressions and see if they are the same. If they are the same, then simply add or subtract the numerators from each other, leaving the denominator alone. If the two denominators are different, however, then you will need to use the above strategy of finding the least common denominator.

When we add or subtract rational expressions, we will not simply be considering the prime factors of integers when looking for the least common denominator. Rather, we will be looking for monomial and binomial factors that are common to both rational expressions. This requires factoring algebraic expressions. 

For example, consider the expression $2x^2 + 4$. Note that each term of the expression is divisible by 2; therefore, this can be rewritten by dividing 2 out of each term: $(2)(x^2 + 2)$. This expression therefore has two factors: $2$ and $(x^2 + 2)$.

Follow the example below to see how this applies to solving addition and subtraction problems.

Example

Subtract the following rational expressions:

$\displaystyle \frac 3 {x^2y+2y}-\frac {4x}{x^2 + 2}$

We begin problems of this type by factoring. Notice that we can rewrite the first denominator in terms of its factors. We can pull $y$ out of the binomial, since it appears in both terms: 

$x^2y+2y = y(x^2 + 2)$

The denominator in the second fraction cannot be factored. The rational expressions therefore become:

$\displaystyle \frac 3 {y(x^2 + 2)} - \frac {4x}{x^2 + 2}$

Notice the factors in the denominators. The first fraction has two factors: $y$ and $(x^2+2)$. The second fraction has only one factor: $(x^2 + 2)$.

Now, as above, we need to find the smallest possible overlap including all the factors in both of these denominators. Through this logic, the least common denominator must have one $(x^2+2)$ and one $y$. 

We now rewrite both fractions with the common denominator (remember that if you multiply a denominator by a factor, you must also multiply the numerator of that fraction by the same factor):

$\displaystyle \frac {3}{y(x^2+2)} - \frac {4x} {x^2+2} \cdot \dfrac{y}{y}$

$\displaystyle \frac {3}{y(x^2+2)} - \frac {4x(y)} {y(x^2+2)}$

Subtracting fractions is easy once you have a common denominator! Now we can simply subtract the numerators:

$\displaystyle \frac {3-4xy} {y(x^2+2)}$

Conclusions

First, always factor rational expressions before doing anything else.

Second, follow the regular procedure for fractions, which in this case involves finding a common denominator. 

Third, subtract the numerators while leaving the denominator alone.

Finally, simplify.