The gradient theorem, also known as the fundamental theorem of calculus for line integrals, says that a line integral through a gradient field can be evaluated by evaluating the original scalar field at the endpoints of the curve.
Let
It is a generalization of the fundamental theorem of calculus to any curve in a plane or space (generally
The gradient theorem implies that line integrals through irrotational vector fields are path-independent. In physics this theorem is one of the ways of defining a "conservative force." By placing
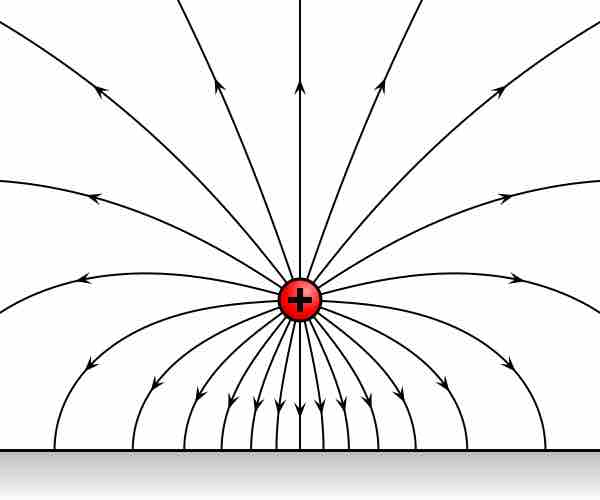
Electric Field Lines of a Positive Charge
Electric field lines emanating from a point where positive electric charge is suspended over a negatively charged infinite sheet. Electric field is a vector field which can be represented as a gradient of a scalar field, called electric potential. Therefore, electric force is a conservative force.
The gradient theorem also has an interesting converse: any conservative vector field can be expressed as the gradient of a scalar field. Just like the gradient theorem itself, this converse has many striking consequences and applications in both pure and applied mathematics
Proof
If
Now suppose the domain
where the definition of the line integral is used in the first equality and the fundamental theorem of calculus is used in the third equality.