The integral test for convergence is a method used to test infinite series of non-negative terms for convergence. It was developed by Colin Maclaurin and Augustin-Louis Cauchy and is sometimes known as the Maclaurin–Cauchy test.
Statement of the test
Consider an integer
Although we won't go into the details, the proof of the test also gives the lower and upper bounds:
for the infinite series.
Applications
The harmonic series
On the other hand, the series
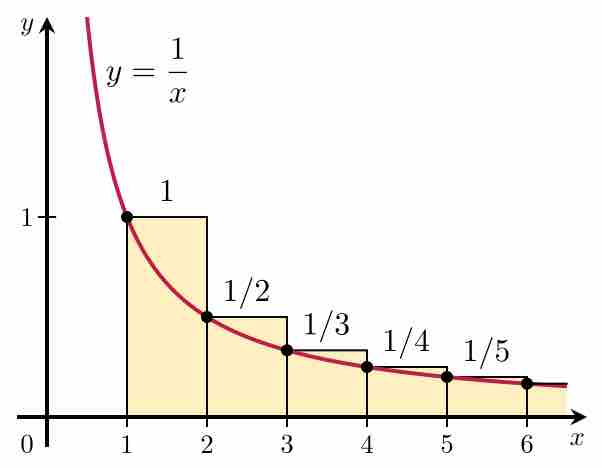
Integral Test
The integral test applied to the harmonic series. Since the area under the curve
The above examples involving the harmonic series raise the question of whether there are monotone sequences such that
and:
for every