The Expanded Octet

Main group elements in the third period and below form compounds that deviate from the octet rule by having more than 8 valence electrons.

Learning Objective

Explain why some elements can form an expanded octet

Key Points

Main group elements that form more bonds than would be predicted by the octet rule are called hypervalent compounds, and have what is known as an 'expanded octet,' meaning that there are more than eight electrons around one atom.
The octet rule can be 'expanded' by some elements by utilizing the d-orbitals found in the third principal energy level and beyond. Sulfur, phosphorus, silicon, and chlorine are common examples of elements that form an expanded octet.
Phosphorus pentachloride (PCl₅) and sulfur hexafluoride (SF₆) are examples of molecules that deviate from the octet rule by having more than 8 electrons around the central atom.

Terms

hypervalent molecule
A molecule that contains an atom from a main group element which deviates from the octet rule by sharing more than eight electrons.
expanded octet
A case where an atom shares more than eight electrons with its bonding partners.
main group element
Elements that are not part of the transition metal block in the periodic table.

Full Text

Deviations from the Octet Rule

A hypervalent molecule is a molecule that contains one or more main group elements that bear more than eight electrons in their valence levels as a result of bonding. Phosphorus pentachloride (PCl₅), sulfur hexafluoride (SF₆), chlorine trifluoride (ClF₃), and the triiodide ion (I₃⁻) are examples of hypervalent molecules.

For the elements in the second period of the periodic table (principal energy level n=2), the s²p⁶ electrons comprise the octet, and no d sublevel exists. As a result, the second period elements (more specifically, the nonmetals C, N, O, F) obey the octet rule without exceptions.

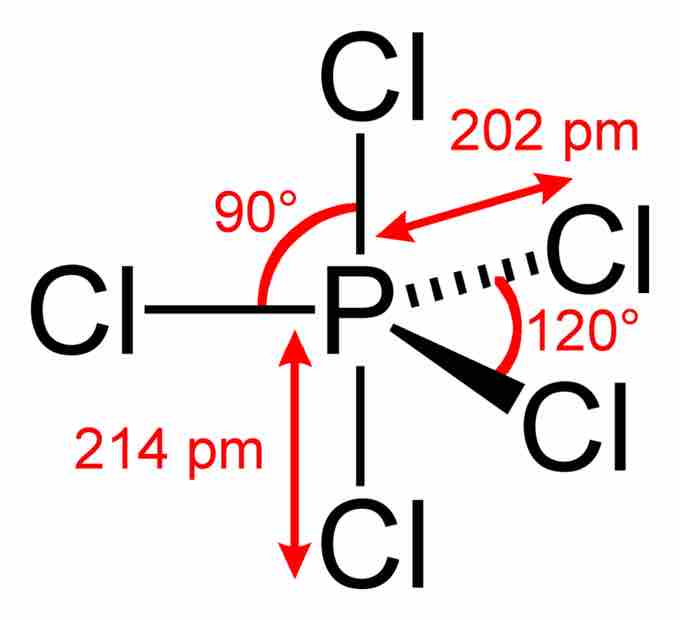
Phosphorus pentachloride

In the PCl₅molecule, the central phosphorus atom is bonded to five Cl atoms, thus having 10 bonding electrons and violating the octet rule. The overall geometry of the molecule is depicted (trigonal bipyramidal), and bond angles and lengths are highlighted.

However, some of the third-period elements (Si, P, S, and Cl) have been observed to bond to more than four other atoms, and thus need to involve more than the four pairs of electrons available in an s²p⁶ octet. This is possible because for n=3, the d sublevel exists, and it has five d orbitals. Although the energy of empty 3d-orbitals is ordinarily higher than that of the 4s orbital, that difference is small and the additional d orbitals can accommodate more electrons. Therefore, the d orbitals participate in bonding with other atoms and an expanded octet is produced. Examples of molecules in which a third period central atom contains an expanded octet are the phosphorus pentahalides and sulfur hexafluoride.

Sulfur hexafluoride

In the SF₆ molecule, the central sulfur atom is bonded to six fluorine atoms, so sulfur has 12 bonding electrons around it. The overall geometry of the molecule is depicted (tetragonal bipyramidal, or octahedral), and bond angles and lengths are highlighted.

For atoms in the fourth period and beyond, higher d orbitals can be used to accommodate additional shared pairs beyond the octet. The relative energies of the different kinds of atomic orbital reveal that energy gaps become smaller as the principal energy level quantum number (n) increases, and the energetic cost of using these higher orbitals to accommodate bonding electrons becomes smaller.

[ edit ]

Prev Concept

Odd-Electron Molecules

Bond Energy

Next Concept