Length Contraction

Length contraction is the physical phenomenon of a decrease in length detected by an observer of objects that travel at any non-zero velocity relative to that observer. Length contraction arises due to the fact that the speed of light in a vacuum is constant in any frame of reference. By taking this into account, as well as some geometrical considerations, we will show how perceived time and length are affected.

Example 1

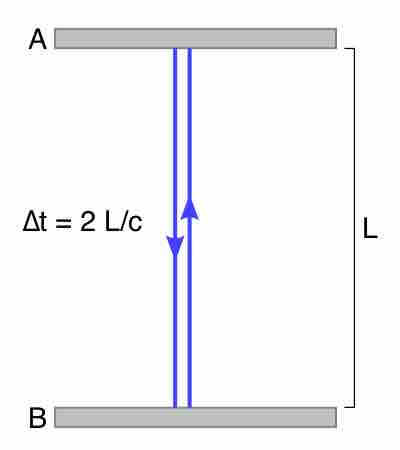
Let us imagine a simple clock system that consists of two mirrors A and B in a vacuum. A light pulse bounces between the two mirrors. The separation of the mirrors is L, and the clock ticks once each time the light pulse hits a given mirror. Now imagine that the clock is at rest. The time that it will take for the light pulse to go from mirror A to mirror B and then back to mirror A be can be described by:

$\Delta t = \frac{2 L}{c}$

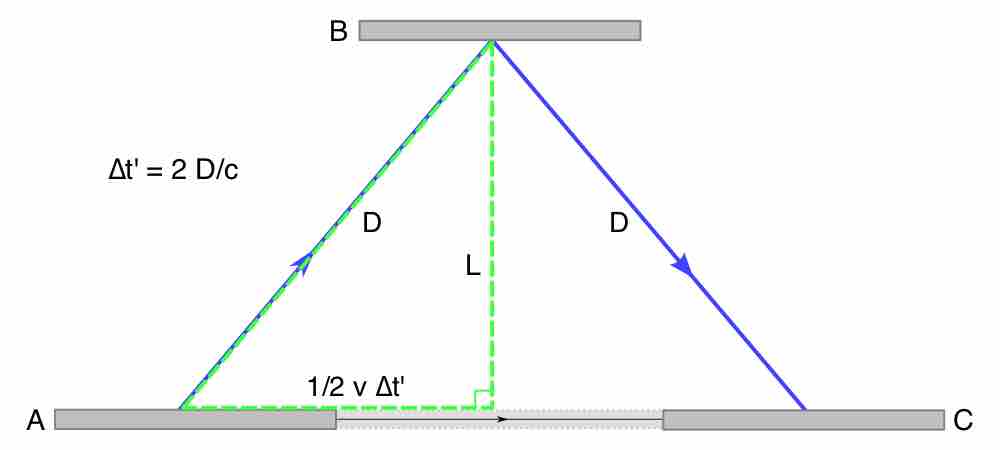
where c is the speed of light . Now imagine that the clock is moving in the horizontal direction relative to a stationary observer. The light pulse is emitted from mirror A. To the stationary observer, it appears that the light pulse has a longer path to travel because by the time the light reaches mirror B the clock has already moved somewhat in the horizontal direction. This is the same case for the light pulse on its way back. The stationary observer will perceive that it will take the light a total of:

Geometry for a Clock at Rest

This illustrates the path that light must traverse when the clock is at rest.

$\Delta t' = \frac{2 D}{c}$

to traverse its path . We can see that D is longer than L, so that means that .

Geometry for a Moving Clock

This illustrates the path that light must traverse when the clock is moving from the perspective of a stationary observer.

Example 2

We have established that in a frame of reference that is moving relative to the clock (the stationary observer is moving in the clock's frame of reference), the clock appears to run more slowly. Now let us imagine that we want to measure the length of a ruler. This time let us imagine that you are moving with velocity v. You can mathematically determine the length of the ruler in your frame of reference (L') by multiplying your velocity (v) by the time that you perceive that it takes you to pass by the ruler (t'). Expressing this in equation form, L' = vt'. Now, if someone in the ruler's rest frame wanted to determine the length of the ruler, they could do the following. They could mathematically determine the length of the ruler in their frame of reference (L) by multiplying your velocity (v) by the time that they perceive that it takes you to pass by the ruler (t). This is expressed in the following equation: L = vt. Just as in the clock explanation, the ruler appears to be moving in your frame of reference, so t will be longer than t' (your time interval). Consequently, the length of the ruler will appear to be shorter in your frame of reference (the phenomenon of length contraction occurred).

The effect of length contraction is negligible at everyday speeds and can be ignored for all regular purposes. Length contraction becomes noticeable at a substantial fraction of the speed of light (as illustrated in ) with the contraction only in the direction parallel to the direction in which the observed body is travelling.

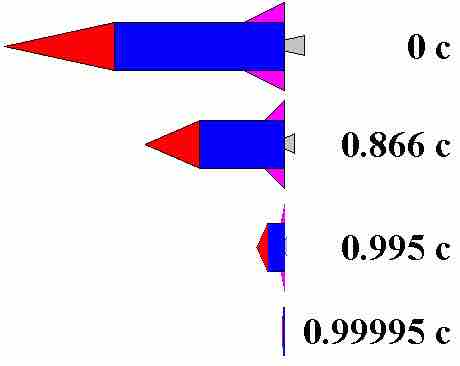
Observed Length of an Object

Observed length of an object at rest and at different speeds

Example 3

For example, at a speed of 13,400,000 m/s (30 million mph, .0447c), the length is 99.9 percent of the length at rest; at a speed of 42,300,000 m/s (95 million mph, 0.141c), the length is still 99 percent. As the magnitude of the velocity approaches the speed of light, the effect becomes dominant.The mathematical formula for length contraction is:

$L = \frac{L_{0}}{\gamma (\upsilon )} = L_{0}\sqrt{1-\frac{\upsilon ^{2}}{c^{2}}}$

where L₀ is the proper length (the length of the object in its rest frame); L is the length observed by an observer in relative motion with respect to the object; v is the relative velocity between the observer and the moving object; c is the speed of light; and the Lorentz factor is defined as:

$\gamma (\upsilon ) = \frac{1}{\sqrt{1 - v^{^{2}}/c^{2}}}$

In this equation it is assumed that the object is parallel with its line of movement. For the observer in relative movement, the length of the object is measured by subtracting the simultaneously measured distances of both ends of the object. An observer at rest viewing an object traveling very close to the speed of light would observe the length of the object in the direction of motion as very close to zero.