Assouad dimension

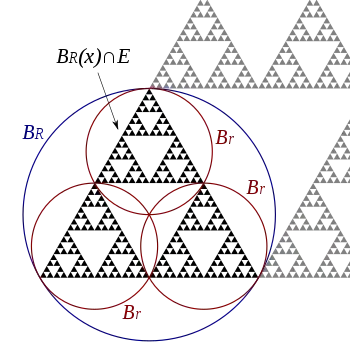
The Assouad dimension of ${\displaystyle X,d_{A}(X)}$, is the infimum of all ${\displaystyle s}$ such that ${\displaystyle (X,\varsigma )}$ is ${\displaystyle (M,s)}$-homogeneous for some ${\displaystyle M\geq 1}$.[3]

Let ${\displaystyle (X,d)}$ be a metric space, and let E be a non-empty subset of X. For r > 0, let ${\displaystyle N_{r}(E)}$ denote the least number of metric open balls of radius less than or equal to r with which it is possible to cover the set E. The Assouad dimension of E is defined to be the infimal ${\displaystyle \alpha \geq 0}$ for which there exist positive constants C and ${\displaystyle \rho }$ so that, whenever

$${\displaystyle 0<r<R\leq \rho ,}$$

the following bound holds:

$${\displaystyle \sup _{x\in E}N_{r}(B_{R}(x)\cap E)\leq C\left({\frac {R}{r}}\right)^{\alpha }.}$$

The intuition underlying this definition is that, for a set E with "ordinary" integer dimension n, the number of small balls of radius r needed to cover the intersection of a larger ball of radius R with E will scale like (R/r)ⁿ.

• The Assouad dimension of a metric space is always greater than or equal to its Assouad–Nagata dimension.[4]
• The Assouad dimension of a metric space is always greater than or equal to its upper box dimension, which in turn is greater than or equal to the Hausdorff dimension.[5]
• The Lebesgue covering dimension of a metrizable space X is the minimal Assouad dimension of any metric on X. In particular, for every metrizable space there is a metric for which the Assouad dimension is equal to the Lebesgue covering dimension.[5]

• Fraser, Jonathan M. (2020). Assouad Dimension and Fractal Geometry. Cambridge University Press. doi:10.1017/9781108778459. ISBN 9781108478656. S2CID 218571013.