Hyperuniformity
Hyperuniform materials are characterized by an anomalous suppression of density fluctuations at large scales. More precisely, the vanishing of density fluctuations in the long-wave length limit (like for crystals) distinguishes hyperuniform systems from typical gases, liquids, or amorphous solids.[1][2] Examples of hyperuniformity include all perfect crystals,[1] perfect quasicrystals,[3][4] and exotic amorphous states of matter.[2]
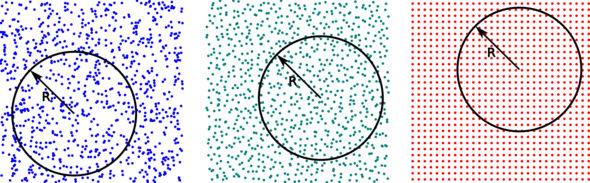
Quantitatively, a many-particle system is said to be hyperuniform if the variance of the number of points within a spherical observation window grows more slowly than the volume of the observation window. This definition is equivalent to a vanishing of the structure factor in the long-wavelength limit,[1] and it has been extended to include heterogeneous materials as well as scalar, vector, and tensor fields.[5] Disordered hyperuniform systems, were shown to be poised at an "inverted" critical point.[1] They can be obtained via equilibrium or nonequilibrium routes, and are found in both classical physical and quantum-mechanical systems.[1][2] Hence, the concept of hyperuniformity now connects a broad range of topics in physics,[2][6][7][8][9] mathematics,[10][11][12][13][14][15] biology,[16][17][18] and materials science.[19][20][21]
The concept of hyperuniformity generalizes the traditional notion of long-range order and thus defines an exotic state of matter. A disordered hyperuniform many-particle system can be statistically isotropic like a liquid, with no Bragg peaks and no conventional type of long-range order. Nevertheless, at large scales, hyperuniform systems resemble crystals, in their suppression of large-scale density fluctuations. This unique combination is known to endow disordered hyperuniform materials with novel physical properties that are, e.g., both nearly optimal and direction independent (in contrast to those of crystals that are anisotropic).[2]
History
The term hyperuniformity (also independently called super-homogeneity in the context of cosmology[22]) was coined and studied by Salvatore Torquato and Frank Stillinger in a 2003 paper,[1] in which they showed that, among other things, hyperuniformity provides a unified framework to classify and structurally characterize crystals, quasicrystals, and exotic disordered varieties. In that sense, hyperuniformity is a long-range property that can be viewed as generalizing the traditional notion of long-range order (e.g., translational / orientational order of crystals or orientational order of quasicrystals) to also encompass exotic disordered systems.[2]
Hyperuniformity was first introduced for point processes[1] and later generalized to two-phase materials (or porous media)[3] and random scalar or vectors fields.[5] It has been observed in theoretical models, simulations, and experiments, see list of examples below.[2]
Definition
A many-particle system in -dimensional Euclidean space is said to be hyperuniform if the number of points in a spherical observation window with radius has a variance that scales slower than the volume of the observation window:[1]
This definition is (essentially) equivalent to the vanishing of the structure factor at the origin:[1]
for wave vectors .
Similarly, a two-phase medium consisting of a solid and a void phase is said to be hyperuniform if the volume of the solid phase inside the spherical observation window has a variance that scales slower than the volume of the observation window. This definition is, in turn, equivalent to a vanishing of the spectral density at the origin.[3]
An essential feature of hyperuniform systems is their scaling of the number variance for large radii or, equivalently, of the structure factor for small wave numbers. If we consider hyperuniform systems that are characterized by a power-law behavior of the structure factor close to the origin:[2]
with a constant , then there are three distinct scaling behaviors that define three classes of hyperuniformity:
Examples are known for all three classes of hyperuniformity.[2]
Examples
Examples of disordered hyperuniform systems in physics are disordered ground states,[7] jammed disordered sphere packings,[6][23][24][25][26][27][28][29][30] amorphous ices,[31] amorphous speckle patterns,[32] certain fermionic systems,[33] random self-organization,[8][34] [35][36][37][38][9] perturbed lattices,[39][40][41][42] and avian photoreceptor cells.[16]
In mathematics, disordered hyperuniformity has been studied in the context of probability theory,[10][43][11] geometry,[13][14] and number theory,[44][12][45] where the prime numbers have been found to be effectively limit periodic and hyperuniform in a certain scaling limit.[12] Further examples include certain random walks[46] and stable matchings of point processes.[15][24][25][26][27][47]
Ordered hyperuniformity
Examples of ordered, hyperuniform systems include all crystals,[1] all quasicrystals,[3][4][48] and limit-periodic sets.[49] While weakly correlated noise typically preserves hyperuniformity, correlated excitations at finite temperature tend to destroy hyperuniformity.[50]
Hyperuniformity was also reported for fermionic quantum matter in correlated electron systems as a result of cramming.[51]
Disordered hyperuniformity
Torquato (2014)[52] gives an illustrative example of the hidden order found in a "shaken box of marbles",[52] which fall into an arrangement, called maximally random jammed packing.[6][53] Such hidden order may eventually be used for self-organizing colloids or optics with the ability to transmit light with an efficiency like a crystal but with a highly flexible design.[52]
It has been found that disordered hyperuniform systems possess unique optical properties. For example, disordered hyperuniform photonic networks have been found to exhibit complete photonic band gaps that are comparable in size to those of photonic crystals but with the added advantage of isotropy, which enables free-form waveguides not possible with crystal structures.[19][20][54][55] Moreover, in stealthy hyperuniform systems,[7] light of any wavelength longer than a value specific to the material is able to propagate forward without loss (due to the correlated disorder) even for high particle density.[56]
By contrast, in conditions where light is propagated through an uncorrelated, disordered material of the same density, the material would appear opaque due to multiple scattering. “Stealthy” hyperuniform materials can be theoretically designed for light of any wavelength, and the applications of the concept cover a wide variety of fields of wave physics and materials engineering.[56][57]
Disordered hyperuniformity was found in the photoreceptor cell patterns in the eyes of chickens.[16] This is thought to be the case because the light-sensitive cells in chicken or other bird eyes cannot easily attain an optimal crystalline arrangement but instead form a disordered configuration that is as uniform as possible.[16][58][59] Indeed, it is the remarkable property of "mulithyperuniformity" of the avian cone patterns, that enables birds to achieve acute color sensing.[16]
Disordered hyperuniformity was recently discovered in amorphous 2‑D materials, which was shown to enhance electronic transport in the material.[60] It may also emerge in the mysterious biological patterns known as fairy circles - circle and patterns of circles that emerge in arid places.[61][62]
Making disordered, but highly uniform, materials
The challenge of creating disordered hyperuniform materials is partly attributed to the inevitable presence of imperfections, such as defects and thermal fluctuations. For example, the fluctuation-compressibility relation dictates that any compressible one-component fluid in thermal equilibrium cannot be strictly hyperuniform at finite temperature.[2]
Recently Chremos & Douglas (2018) proposed a design rule for the practical creation of hyperuniform materials at the molecular level.[63][64] Specifically, effective hyperuniformity as measured by the hyperuniformity index is achieved by specific parts of the molecules (e.g., the core of the star polymers or the backbone chains in the case of bottlebrush polymers).[65][2]
The combination of these features leads to molecular packings that are highly uniform at both small and large length scales.[63][64]
Non-equilibrium hyperuniform fluids and length scales
Disordered hyperuniformity implies a long-ranged direct correlation function (the Ornstein–Zernike equation).[1] In an equilibrium many-particle system, this requires delicately designed effectively long-ranged interactions, which are not necessary for the dynamic self-assembly of non-equilibrium hyperuniform states. In 2019, Ni and co-workers theoretically predicted a non-equilibrium strongly hyperuniform fluid phase that exists in systems of circularly swimming active hard spheres,[34] which was confirmed experimentally in 2022.[66]
This new hyperuniform fluid features a special length scale, i.e., the diameter of the circular trajectory of active particles, below which large density fluctuations are observed. Moreover, based on a generalized random organising model, Lei and Ni (2019)[35] formulated a hydrodynamic theory for non-equilibrium hyperuniform fluids, and the length scale above which the system is hyperuniform is controlled by the inertia of the particles. The theory generalizes the mechanism of fluidic hyperuniformity as the damping of the stochastic harmonic oscillator, which indicates that the suppressed long-wavelength density fluctuation can exhibit as either acoustic (resonance) mode or diffusive (overdamped) mode.[35]
References
- Torquato, Salvatore; Stillinger, Frank H. (29 October 2003). "Local density fluctuations, hyperuniformity, and order metrics". Physical Review E. 68 (4): 041113. arXiv:cond-mat/0311532. Bibcode:2003PhRvE..68d1113T. doi:10.1103/PhysRevE.68.041113. PMID 14682929. S2CID 9162488.
- Torquato, Salvatore (2018). "Hyperuniform states of matter". Physics Reports. 745: 1–95. arXiv:1801.06924. Bibcode:2018PhR...745....1T. doi:10.1016/j.physrep.2018.03.001. S2CID 119378373.
- Zachary, Chase E.; Torquato, Salvatore (21 December 2009). "Hyperuniformity in point patterns and two-phase random heterogeneous media". Journal of Statistical Mechanics: Theory and Experiment. 2009 (12): P12015. arXiv:0910.2172. Bibcode:2009JSMTE..12..015Z. doi:10.1088/1742-5468/2009/12/P12015. ISSN 1742-5468. S2CID 18838058.
- Oğuz, Erdal C.; Socolar, Joshua E.S.; Steinhardt, Paul J.; Torquato, Salvatore (23 February 2017). "Hyperuniformity of quasicrystals". Physical Review B. 95 (5): 054119. arXiv:1612.01975. Bibcode:2017PhRvB..95e4119O. doi:10.1103/PhysRevB.95.054119. ISSN 2469-9950. S2CID 85522310.
- Torquato, Salvatore (15 August 2016). "Hyperuniformity and its generalizations". Physical Review E. 94 (2): 022122. arXiv:1607.08814. Bibcode:2016PhRvE..94b2122T. doi:10.1103/PhysRevE.94.022122. ISSN 2470-0045. PMID 27627261. S2CID 30459937.
- Donev, Aleksandar; Stillinger, Frank H.; Torquato, Salvatore (26 August 2005). "Unexpected density fluctuations in jammed disordered sphere packings". Physical Review Letters. 95 (9): 090604. arXiv:cond-mat/0506406. Bibcode:2005PhRvL..95i0604D. doi:10.1103/PhysRevLett.95.090604. ISSN 0031-9007. PMID 16197201. S2CID 7887194.
- Torquato, S.; Zhang, G.; Stillinger, F.H. (29 May 2015). "Ensemble theory for stealthy hyperuniform disordered ground states". Physical Review X. 5 (2): 021020. arXiv:1503.06436. Bibcode:2015PhRvX...5b1020T. doi:10.1103/PhysRevX.5.021020. ISSN 2160-3308. S2CID 17275490.
- Hexner, Daniel; Levine, Dov (20 March 2015). "Hyperuniformity of Critical Absorbing States". Physical Review Letters. 114 (11): 110602. arXiv:1407.0146. Bibcode:2015PhRvL.114k0602H. doi:10.1103/PhysRevLett.114.110602. ISSN 0031-9007. PMID 25839254. S2CID 23951607.
- Wilken, Sam; Guerra, Rodrigo E.; Pine, David J.; Chaikin, Paul M. (11 February 2020). "Hyperuniform Structures Formed by Shearing Colloidal Suspensions". Physical Review Letters. 125 (14): 148001. arXiv:2002.04499. Bibcode:2020PhRvL.125n8001W. doi:10.1103/PhysRevLett.125.148001. PMID 33064537. S2CID 211075881.
- Ghosh, Subhroshekhar; Lebowitz, Joel L. (2017). "Fluctuations, large deviations and rigidity in hyperuniform systems: A brief survey". Indian Journal of Pure and Applied Mathematics. 48 (4): 609–631. arXiv:1608.07496. doi:10.1007/s13226-017-0248-1. ISSN 0019-5588. S2CID 8709357.
- Ghosh, Subhroshekhar; Lebowitz, Joel L. (2018). "Generalized stealthy hyperuniform processes: Maximal rigidity and the bounded holes conjecture". Communications in Mathematical Physics. 363 (1): 97–110. arXiv:1707.04328. Bibcode:2018CMaPh.363...97G. doi:10.1007/s00220-018-3226-5. ISSN 0010-3616. S2CID 6243545.
- Torquato, Salvatore; Zhang, Ge; De Courcy-Ireland, Matthew (29 March 2019). "Hidden multiscale order in the primes". Journal of Physics A: Mathematical and Theoretical. 52 (13): 135002. arXiv:1804.06279. Bibcode:2019JPhA...52m5002T. doi:10.1088/1751-8121/ab0588. ISSN 1751-8113. S2CID 85508362.
- Brauchart, Johann S.; Grabner, Peter J.; Kusner, Wöden; Ziefle, Jonas (2020). "Hyperuniform point sets on the sphere: probabilistic aspects". Monatshefte für Mathematik. 192 (4): 763–781. arXiv:1809.02645. doi:10.1007/s00605-020-01439-y. ISSN 0026-9255. S2CID 119179807.
- Baake, Michael; Grimm, Uwe (1 September 2020). "Inflation versus projection sets in aperiodic systems: The role of the window in averaging and diffraction". Acta Crystallographica Section A. 76 (5): 559–570. arXiv:2004.03256. doi:10.1107/S2053273320007421. ISSN 2053-2733. PMC 7459767. PMID 32869753. S2CID 220404667.
- Klatt, Michael Andreas; Last, Günter; Yogeshwaran, D. (2020). "Hyperuniform and rigid stable matchings". Random Structures & Algorithms. 57 (2): 439–473. arXiv:1810.00265. doi:10.1002/rsa.20923. ISSN 1098-2418. S2CID 119678948.
- Jiao; et al. (2014). "Avian Photoreceptor Patterns Represent a Disordered Hyperuniform Solution to a Multiscale Packing Problem". Physical Review E. 89 (2): 022721. arXiv:1402.6058. Bibcode:2014PhRvE..89b2721J. doi:10.1103/PhysRevE.89.022721. PMC 5836809. PMID 25353522.
- Mayer, Andreas; Balasubramanian, Vijay; Mora, Thierry; Walczak, Aleksandra M. (12 May 2015). "How a well-adapted immune system is organized". Proceedings of the National Academy of Sciences. 112 (19): 5950–5955. arXiv:1407.6888. Bibcode:2015PNAS..112.5950M. doi:10.1073/pnas.1421827112. ISSN 0027-8424. PMC 4434741. PMID 25918407.
- Huang, Mingji; Hu, Wensi; Yang, Siyuan; Liu, Quan-Xing; Zhang, H. P. (4 May 2021). "Circular swimming motility and disordered hyperuniform state in an algae system". Proceedings of the National Academy of Sciences. 118 (18): e2100493118. Bibcode:2021PNAS..11800493H. doi:10.1073/pnas.2100493118. ISSN 0027-8424. PMC 8106356. PMID 33931505.
- Florescu, M.; Torquato, S.; Steinhardt, P.J. (8 December 2009). "Designer disordered materials with large, complete photonic band gaps". Proceedings of the National Academy of Sciences. 106 (49): 20658–20663. arXiv:1007.3554. Bibcode:2009PNAS..10620658F. doi:10.1073/pnas.0907744106. ISSN 0027-8424. PMC 2777962. PMID 19918087.
- Muller, Nicolas; Haberko, Jakub; Marichy, Catherine; Scheffold, Frank (2014). "Silicon hyperuniform disordered photonic materials with a pronounced gap in the shortwave infrared" (PDF). Advanced Optical Materials. 2 (2): 115–119. doi:10.1002/adom.201300415.
- Yu, Sunkyu (13 February 2023). "Evolving scattering networks for engineering disorder". Nature Computational Science. 3 (2): 128–138. doi:10.1038/s43588-022-00395-x. ISSN 2662-8457. S2CID 256862885.
- Gabrielli, Andrea; Joyce, Michael; Sylos Labini, Francesco (11 April 2002). "Glass-like universe: Real-space correlation properties of standard cosmological models". Physical Review D. 65 (4): 083523. arXiv:astro-ph/0110451. Bibcode:2002PhRvD..65h3523G. doi:10.1103/PhysRevD.65.083523. PMID 14682929. S2CID 9162488.
- Zachary, Chase E.; Jiao, Yang; Torquato, Salvatore (29 April 2011). "Hyperuniform long-range correlations are a signature of disordered jammed hard-particle packings". Physical Review Letters. 106 (17): 178001. arXiv:1008.2548. Bibcode:2011PhRvL.106q8001Z. doi:10.1103/PhysRevLett.106.178001. ISSN 0031-9007. PMID 21635063. S2CID 15587068.
- Weijs, Joost H.; Jeanneret, Raphaël; Dreyfus, Rémi; Bartolo, Denis (3 September 2015). "Emergent Hyperuniformity in Periodically Driven Emulsions". Physical Review Letters. 115 (10): 108301. arXiv:1504.04638. Bibcode:2015PhRvL.115j8301W. doi:10.1103/PhysRevLett.115.108301. ISSN 0031-9007. PMID 26382706. S2CID 10340709.
- Jack, Robert L.; Thompson, Ian R.; Sollich, Peter (9 February 2015). "Hyperuniformity and Phase Separation in Biased Ensembles of Trajectories for Diffusive Systems". Physical Review Letters. 114 (6): 060601. arXiv:1409.3986. Bibcode:2015PhRvL.114f0601J. doi:10.1103/PhysRevLett.114.060601. ISSN 0031-9007. PMID 25723197. S2CID 3132460.
- Weijs, Joost H.; Bartolo, Denis (27 July 2017). "Mixing by Unstirring: Hyperuniform Dispersion of Interacting Particles upon Chaotic Advection". Physical Review Letters. 119 (4): 048002. arXiv:1702.02395. Bibcode:2017PhRvL.119d8002W. doi:10.1103/PhysRevLett.119.048002. ISSN 0031-9007. PMID 29341775. S2CID 12229553.
- Ricouvier, Joshua; Pierrat, Romain; Carminati, Rémi; Tabeling, Patrick; Yazhgur, Pavel (15 November 2017). "Optimizing Hyperuniformity in Self-Assembled Bidisperse Emulsions". Physical Review Letters. 119 (20): 208001. arXiv:1711.00719. Bibcode:2017PhRvL.119t8001R. doi:10.1103/PhysRevLett.119.208001. ISSN 0031-9007. PMID 29219379. S2CID 28177098.
- Chieco, A. T.; Zu, M.; Liu, A. J.; Xu, N.; Durian, D. J. (17 October 2018). "Spectrum of structure for jammed and unjammed soft disks". Physical Review E. 98 (4): 042606. arXiv:1806.10118. Bibcode:2018PhRvE..98d2606C. doi:10.1103/PhysRevE.98.042606. ISSN 2470-0045. S2CID 119448635.
- Wilken, Sam; Guerra, Rodrigo E.; Levine, Dov; Chaikin, Paul M. (12 July 2021). "Random Close Packing as a Dynamical Phase Transition". Physical Review Letters. 127 (3): 038002. Bibcode:2021PhRvL.127c8002W. doi:10.1103/PhysRevLett.127.038002. ISSN 0031-9007. OSTI 1850634. PMID 34328779. S2CID 236531841.
- Rissone, Paolo; Corwin, Eric I.; Parisi, Giorgio (12 July 2021). "Long-Range Anomalous Decay of the Correlation in Jammed Packings". Physical Review Letters. 127 (3): 038001. arXiv:2012.10181. Bibcode:2021PhRvL.127c8001R. doi:10.1103/PhysRevLett.127.038001. ISSN 0031-9007. PMID 34328763. S2CID 229331981.
- Martelli, Fausto; Torquato, Salvatore; Giovambattista, Nicolas; Car, Roberto (29 September 2017). "Large-Scale Structure and Hyperuniformity of Amorphous Ices". Physical Review Letters. 119 (13): 136002. arXiv:1705.09961. Bibcode:2017PhRvL.119m6002M. doi:10.1103/PhysRevLett.119.136002. PMID 29341697. S2CID 44864111.
- Di Battista, Diego; Ancora, Daniele; Zacharakis, Giannis; Ruocco, Giancarlo; Leonetti, Marco (11 June 2018). "Hyperuniformity in amorphous speckle patterns". Optics Express. 26 (12): 15594–15608. arXiv:1803.09550. Bibcode:2018OExpr..2615594D. doi:10.1364/OE.26.015594. hdl:11311/1142259. ISSN 1094-4087. PMID 30114818. S2CID 52031100.
- Torquato, Salvatore; Scardicchio, A; Zachary, Chase E (27 November 2008). "Point processes in arbitrary dimension from fermionic gases, random matrix theory, and number theory". Journal of Statistical Mechanics: Theory and Experiment. 2008 (11): P11019. arXiv:0809.0449. Bibcode:2008JSMTE..11..019T. doi:10.1088/1742-5468/2008/11/P11019. ISSN 1742-5468. S2CID 6252369.
- Lei, Qunli; Pica Ciamarra, Massimo; Ni, Ran (25 January 2019). "Non-Equilibrium Strongly Hyperuniform Fluids of Circle Active Particles with Large Local Density Fluctuations". Science Advances. 5 (1): eaau7423. arXiv:1802.03682. Bibcode:2019SciA....5.7423L. doi:10.1126/sciadv.aau7423. PMC 6357732. PMID 30746459.
- Lei, Qunli; Ni, Ran (12 November 2019). "Hydrodynamics of random-organizing hyperuniform fluids". Proceedings of the National Academy of Sciences of the United States of America. 116 (46): 22983–22989. arXiv:1904.07514. Bibcode:2019PNAS..11622983L. doi:10.1073/pnas.1911596116. PMC 6859356. PMID 31666326.
- Hexner, Daniel; Chaikin, Paul M.; Levine, Dov (25 April 2017). "Enhanced hyperuniformity from random reorganization". Proceedings of the National Academy of Sciences. 114 (17): 4294–4299. Bibcode:2017PNAS..114.4294H. doi:10.1073/pnas.1619260114. ISSN 0027-8424. PMC 5410804. PMID 28396393.
- Garcia-Millan, R.; Pruessner, G.; Pickering, L.; Christensen, K. (17 July 2018). "Correlations and hyperuniformity in the avalanche size of the Oslo model". EPL (Europhysics Letters). 122 (5): 50003. arXiv:1710.00179. Bibcode:2018EL....12250003G. doi:10.1209/0295-5075/122/50003. ISSN 1286-4854. S2CID 52440880.
- Ness, Christopher; Cates, Michael E. (27 February 2020). "Absorbing-State Transitions in Granular Materials Close to Jamming". Physical Review Letters. 124 (8): 088004. arXiv:2001.10228. Bibcode:2020PhRvL.124h8004N. doi:10.1103/PhysRevLett.124.088004. ISSN 0031-9007. PMID 32167320. S2CID 210932396.
- Gabrielli, Andrea; Joyce, Michael; Sylos Labini, Francesco (11 April 2002). "Glass-like universe: Real-space correlation properties of standard cosmological models". Physical Review D. 65 (8): 083523. arXiv:astro-ph/0110451. Bibcode:2002PhRvD..65h3523G. doi:10.1103/PhysRevD.65.083523. ISSN 0556-2821. S2CID 119442907.
- Gabrielli, Andrea (2004). "Point processes and stochastic displacement fields". Physical Review E. 70 (6): 066131. arXiv:cond-mat/0409594. Bibcode:2004PhRvE..70f6131G. doi:10.1103/PhysRevE.70.066131. ISSN 1539-3755. PMID 15697458. S2CID 33621420.
- Le Thien, Q.; McDermott, D.; Reichhardt, C.J.O.; Reichhardt, C. (15 September 2017). "Enhanced pinning for vortices in hyperuniform pinning arrays and emergent hyperuniform vortex configurations with quenched disorder". Physical Review B. 96 (9): 094516. Bibcode:2017PhRvB..96i4516L. doi:10.1103/PhysRevB.96.094516. ISSN 2469-9950. S2CID 18031713.
- Klatt, Michael A.; Kim, Jaeuk; Torquato, Salvatore (13 March 2020). "Cloaking the underlying long-range order of randomly perturbed lattices". Physical Review E. 101 (3): 032118. arXiv:2001.08161. Bibcode:2020PhRvE.101c2118K. doi:10.1103/PhysRevE.101.032118. ISSN 2470-0045. PMID 32289999. S2CID 210859161.
- Ghosh, Subhro; Lebowitz, Joel (2017). "Number Rigidity in Superhomogeneous Random Point Fields". Journal of Statistical Physics. 166 (3–4): 1016–1027. arXiv:1601.04216. Bibcode:2017JSP...166.1016G. doi:10.1007/s10955-016-1633-6. ISSN 0022-4715. S2CID 19675015.
- Zhang, G; Martelli, F; Torquato, S (16 March 2018). "The structure factor of primes". Journal of Physics A: Mathematical and Theoretical. 51 (11): 115001. arXiv:1801.01541. Bibcode:2018JPhA...51k5001Z. doi:10.1088/1751-8121/aaa52a. ISSN 1751-8113. S2CID 67819480.
- Baake, Michael; Coons, Michael (2021). "Scaling of the Diffraction Measure of $k$ -Free Integers Near the Origin". Michigan Mathematical Journal. 70: 213–221. arXiv:1904.00279. doi:10.1307/mmj/1592877613. ISSN 0026-2285. S2CID 90260746.
- Casini, Emanuele; Le Caër, Gérard; Martinelli, Andrea (2015). "Short Hyperuniform Random Walks" (PDF). Journal of Statistical Physics. 160 (1): 254–273. Bibcode:2015JSP...160..254C. doi:10.1007/s10955-015-1244-7. ISSN 0022-4715. S2CID 45170541.
- Chieco, A.T.; Zu, M.; Liu, A.J.; Xu, N.; Durian, D.J. (17 October 2018). "Spectrum of structure for jammed and unjammed soft disks". Physical Review E. 98 (4): 042606. arXiv:1806.10118. Bibcode:2018PhRvE..98d2606C. doi:10.1103/PhysRevE.98.042606. ISSN 2470-0045. S2CID 119448635.
- Lin, C.; Steinhardt, P.J.; Torquato, S. (13 April 2017). "Hyperuniformity variation with quasicrystal local isomorphism class". Journal of Physics: Condensed Matter. 29 (20): 204003. Bibcode:2017JPCM...29t4003L. doi:10.1088/1361-648x/aa6944. ISSN 0953-8984. PMID 28345537. S2CID 46764513.
- Baake, Michael; Grimm, Uwe (23 May 2019). "Scaling of diffraction intensities near the origin: Some rigorous results". Journal of Statistical Mechanics: Theory and Experiment. 2019 (5): 054003. arXiv:1905.04177. Bibcode:2019JSMTE..05.4003B. doi:10.1088/1742-5468/ab02f2. ISSN 1742-5468.
- Kim, Jaeuk; Torquato, Salvatore (12 February 2018). "Effect of imperfections on the hyperuniformity of many-body systems". Physical Review B. 97 (5): 054105. Bibcode:2018PhRvB..97e4105K. doi:10.1103/PhysRevB.97.054105. ISSN 2469-9950.
- Gerasimenko; et al. (2019). "Quantum jamming transition to a correlated electron glass in 1T-TaS2". Nature Materials. 317 (10): 1078–1083. arXiv:1803.00255. Bibcode:2019NatMa..18.1078G. doi:10.1038/s41563-019-0423-3. PMID 31308513. S2CID 196810837.
- Kelly, Morgan (24 February 2014). "In the eye of a chicken, a new state of matter comes into view" (Press release). Princeton, NJ: Princeton University. Retrieved 8 March 2021.
- Atkinson, Steven; Stillinger, Frank H.; Torquato, Salvatore (30 December 2014). "Existence of isostatic, maximally random jammed monodisperse hard-disk packings". Proceedings of the National Academy of Sciences. 111 (52): 18436–18441. Bibcode:2014PNAS..11118436A. doi:10.1073/pnas.1408371112. ISSN 0027-8424. PMC 4284597. PMID 25512529.
- Froufe-Pérez, Luis S.; Engel, Michael; Sáenz, Juan José; Scheffold, Frank (5 September 2017). "Band gap formation and Anderson localization in disordered photonic materials with structural correlations". Proceedings of the National Academy of Sciences. 114 (36): 9570–9574. arXiv:1702.03883. Bibcode:2017PNAS..114.9570F. doi:10.1073/pnas.1705130114. ISSN 0027-8424. PMC 5594660. PMID 28831009.
- Milošević, Milan M.; Man, Weining; Nahal, Geev; Steinhardt, Paul J.; Torquato, Salvatore; Chaikin, Paul M.; Amoah, Timothy; Yu, Bowen; Mullen, Ruth Ann; Florescu, Marian (2019). "Hyperuniform disordered waveguides and devices for near infrared silicon photonics". Scientific Reports. 9 (1): 20338. Bibcode:2019NatSR...920338M. doi:10.1038/s41598-019-56692-5. ISSN 2045-2322. PMC 6937303. PMID 31889165.
- Leseur, O.; Pierrat, R.; Carminati, R. (2016). "High-density hyperuniform materials can be transparent". Optica. 3 (7): 763. arXiv:1510.05807. Bibcode:2016Optic...3..763L. doi:10.1364/OPTICA.3.000763. S2CID 118443561.
- Gorsky, S.; Britton, W. A.; Chen, Y.; Montaner, J.; Lenef, A.; Raukas, M.; Dal Negro, L. (1 November 2019). "Engineered hyperuniformity for directional light extraction". APL Photonics. 4 (11): 110801. Bibcode:2019APLP....4k0801G. doi:10.1063/1.5124302. ISSN 2378-0967.
- Melissa (21 March 2014). "Disordered hyperuniformity: A weird new state of matter in chicken eyes". TodayIFoundOut.com. Gawker Media – via Gizmodo.
- David Freeman (26 February 2014). "Scientists Look In Chicken's Eye And Discover Weird New State Of Matter". The Huffington Post. Retrieved 20 December 2015.
- Yu; et al. (2020). "Disordered hyperuniformity in two-dimensional amorphous silica". Science Advances. 6 (16): eaba0826. Bibcode:2020SciA....6A.826Z. doi:10.1126/sciadv.aba0826. PMC 7164937. PMID 32494625. S2CID 218844271.
- "Dragons, aliens, bugs? Scientists may have solved the mystery of the desert's 'fairy circles'". The Washington Post. 18 January 2017.
The thing that immediately caught my eye about what they had was it seemed to fall into an exotic type of patterning I call hyperuniformity. — Salvatore Torquato
- Getzin, Stephan; et al. (2016). "Discovery of fairy circles in Australia supports self-organization theory1". Proceedings of the National Academy of Sciences. 113 (13): 3551–3556. Bibcode:2016PNAS..113.3551G. doi:10.1073/pnas.1522130113. PMC 4822591. PMID 26976567 – via researchgate.net.
- Chremos, Alexandros; Douglas, Douglas F. (21 December 2018). "Hidden hyperuniformity in soft polymeric materials". Physical Review Letters. 121 (25): 258002. Bibcode:2018PhRvL.121y8002C. doi:10.1103/PhysRevLett.121.258002. PMID 30608782.
- Chremos, Alexandros (7 August 2020). "Design of nearly perfect hyperuniform polymeric materials". The Journal of Chemical Physics. 153 (5): 054902. Bibcode:2020JChPh.153e4902C. doi:10.1063/5.0017861. ISSN 0021-9606. PMC 7530914. PMID 32770903.
- Atkinson, Steven; Zhang, Ge; Hopkins, Adam B.; Torquato, Salvatore (8 July 2016). "Critical slowing down and hyperuniformity on approach to jamming". Physical Review E. 94 (1): 012902. arXiv:1606.05227. Bibcode:2016PhRvE..94a2902A. doi:10.1103/PhysRevE.94.012902. ISSN 2470-0045. PMID 27575201. S2CID 12103288.
- Zhang, Bo; Snezhko, Alexey (27 May 2022). "Hyperuniform Active Chiral Fluids with Tunable Internal Structure". Physical Review Letters. 128 (21): 218002. arXiv:2205.12384. Bibcode:2022PhRvL.128u8002Z. doi:10.1103/PhysRevLett.128.218002. PMID 35687470. S2CID 249063085.
External links
- Wolchover, Natalie. "A bird's-eye view of nature's hidden order". Quanta Magazine.
- Wolchover, Natalie. "A chemist shines light on a surprising prime number pattern". Quanta Magazine.