Dobble

Dobble is a game in which players have to find symbols in common between two cards. It was the UK’s best-selling game in 2018 and 2019.[1][2][3]

For the Ghanian music duo, see Dobble (music duo).
Dobble
Alternative namesSpot It!
TypeMatching
Players2-8
Age range8+
Cards55
DeckProprietary cards
Playing time15 minutes

The game is sold as Dobble in Europe and Spot It! in the US.[4] The name is a play on the word 'double'.[5]

Gameplay

The game uses a deck of 55 cards, each printed with eight different symbols. Any two cards always share one, and only one, matching symbol. The objective of the game is to be the first player to announce the common symbol between two given cards.[4]

Development

In 1976, inspired by Kirkman's schoolgirl problem, French mathematics enthusiast Jacques Cottereau devised a game consisting of a set of 31 cards each with six images of insects, with exactly one image shared between each pair of them. In 2008, journalist and game designer Denis Blanchot found a few of the cards from the "game of insects" and developed the idea to create Dobble.[5]

Dobble was released in France in 2009, and in the UK and North America in 2011 under Blue Orange Games. In 2015, the French board game company Asmodee acquired the rights to Dobble and Spot It!.[5][6][7]

Mathematics
The Fano Plane
Graph of the projective plane of order 7, having 57 points, 57 lines, 8 points on each line and 8 lines passing through each point, where each point is denoted by a rounded rectangle and each line by a combination of letter and number. Only lines with letter A and H are drawn. In the Dobble or Spot It! game, two points are removed. In the SVG file, hover over a line to highlight it.

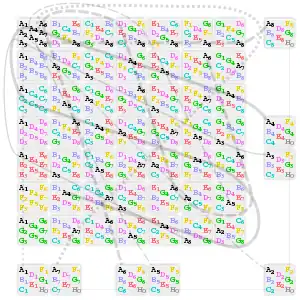
The special way that symbols are arranged on Dobble cards can be understood using geometry. If each card is represented by a line, and each symbol by a point where two lines intersect, then the properties of Dobble are that:

  • any two lines intersect at exactly one point, and
  • any two points are joined by exactly one line.

This geometric structure is an example of a finite projective plane.[8]

If there are 3 points in each line this creates a structure known as the Fano plane. This represents a simpler version of Dobble with 3 symbols on each card, 7 cards and 7 symbols.[8]

In general, a finite projective plane of order n has n+1 points on each line, and n2+n+1 points and lines.[8] This implies that a finite projective plane of order n-1 has n points on each line, and n2-n+1 points and lines.

The real game of Dobble with 8 symbols on each card corresponds to the finite projective plane of order 7, where each line joins 8 points. This results in a structure with 57 lines and 57 points (72+7+1=82-8+1=57),[8] corresponding to 57 cards and 57 symbols. However, the game works fine with fewer cards too, and Dobble is marketed with 55 cards in the deck (but 57 different symbols).[5][9] A junior version of Dobble is marketed with 6 symbols per card, 30 cards, and 31 different symbols (62-6+1=31).

References
  1. "Asmodee named UK's No.1 Games Manufacturer of 2019". Asmodee United Kingdom. Archived from the original on 2020-03-01. Retrieved 2020-03-01.
  2. Smithers, Rebecca (2019-12-15). "Families get on board with fast, easy games for Christmas". The Observer. ISSN 0029-7712. Retrieved 2020-03-01.
  3. "Dobble named UK's biggest-selling card game as one millionth unit sold". ToyNews. Archived from the original on 2020-08-01. Retrieved 2020-03-01.
  4. "Dobble | Card Game". Rules of Play. Retrieved 2020-03-01.
  5. McRobbie, Linda Rodriguez. "The Mind-Bending Math Behind Spot It!, the Beloved Family Card Game". Smithsonian Magazine. Retrieved 2020-03-01.
  6. Asmodee (2015-07-02). "Asmodee acquires the rights to Spot It! Game". Retrieved 2016-01-29.
  7. "Asmodee Acquires the Rights to Spot It! Game". www.businesswire.com. 2015-07-02. Retrieved 2020-03-04.
  8. "Finite Projective Planes and the Math of Spot It!". puzzlewocky. 2016-02-18. Retrieved 2020-03-03.
  9. Polster, Burkard (2015-04-01). "The Intersection Game" (PDF). Math Horizons. 22 (4): 8–11. doi:10.4169/mathhorizons.22.4.8. ISSN 1072-4117. S2CID 125989030. Archived from the original (PDF) on 2020-02-07.
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.