Supergolden ratio

A triangle with side lengths ${\displaystyle \psi }$, 1, and ${\displaystyle \psi ^{-1}}$ has an angle of exactly 120 degrees opposite the side of length ${\displaystyle \psi }$.[2]

Many properties of the supergolden ratio are closely related to golden ratio ${\displaystyle \phi }$. For example, while we have ${\displaystyle \varphi -1=\varphi ^{-1}}$ for the golden ratio, the inverse square of the supergolden ratio obeys ${\displaystyle \psi -1=\psi ^{-2}}$.[3] Additionally, the supergolden ratio can be expressed in terms of itself as the infinite geometric series[3]

${\displaystyle \psi ^{3}=\sum _{n=0}^{\infty }\psi ^{-n},}$

in comparison to the golden ratio identity

${\displaystyle \varphi ^{2}=\sum _{n=0}^{\infty }\varphi ^{-n}.}$

The supergolden ratio is also the fourth smallest Pisot number, which means that its algebraic conjugates are both smaller than 1 in absolute value.[2]

The supergolden sequence, also known as the Narayana's cows sequence, is a sequence where the ratio between consecutive terms approaches the supergolden ratio.[4] The first three terms are each one, and each term after that is calculated by adding the previous term and the term two places before that; that is, ${\displaystyle a_{n+1}=a_{n}+a_{n-2}}$, with ${\displaystyle a_{1}=a_{2}=a_{3}=1}$. The first values are 1, 1, 1, 2, 3, 4, 6, 9, 13, 19, 28, 41, 60, 88, 129, 189, 277, 406, 595…[4][3] ((sequence A000930 in the OEIS)).

This diagram shows the lengths of decreasing powers within a supergolden rectangle, and the pattern of intersecting right angles that appears as a result.

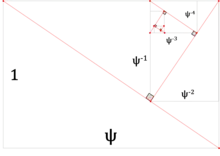
A supergolden rectangle is a rectangle whose side lengths are in a ψ∶1 ratio. When a square with the same side length as the shorter side of the rectangle is removed from one side of the rectangle, the sides of the resulting rectangle will be in a ψ²∶1 ratio. This rectangle can be divided into two more supergolden rectangles with opposite orientations and areas in a ψ²∶1 ratio. The larger rectangle has a diagonal of length ${\displaystyle 1/{\sqrt {\psi }}}$ times the short side of the original rectangle, and which is perpendicular to the diagonal of the original rectangle.[4][3]

In addition, if the line segment that separates the two supergolden rectangles is extended across the square, then each diagonally opposite pair of rectangles has a combined area which is half that of the original rectangle.[1] The larger of the new rectangles is also a supergolden rectangle, with a diagonal of length ${\displaystyle {\sqrt {\psi }}}$ times the length of the short side of the original rectangle;[3] while the smaller one has sides in a ψ³∶1 ratio.[1]

• Solutions to equations similar to ${\displaystyle x^{3}=x^{2}+1}$:
  • Golden ratio – the only positive solution to the equation ${\displaystyle x^{2}=x+1}$
  • Plastic number – the only real solution to the equation ${\displaystyle x^{3}=x+1}$