Tetrahedral molecular geometry

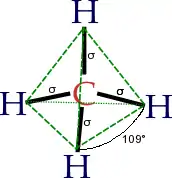
In a tetrahedral molecular geometry, a central atom is located at the center with four substituents that are located at the corners of a tetrahedron. The bond angles are cos−1(−1⁄3) = 109.4712206...° ≈ 109.5° when all four substituents are the same, as in methane (CH4)[1][2] as well as its heavier analogues. Methane and other perfectly symmetrical tetrahedral molecules belong to point group Td, but most tetrahedral molecules have lower symmetry. Tetrahedral molecules can be chiral.

Tetrahedral molecular geometry
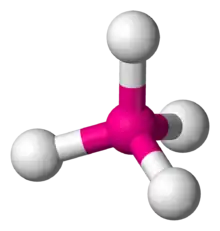ExamplesCH4, MnO−
4
Point groupTd
Coordination number4
Bond angle(s)≈ 109.5°
μ (Polarity)0

Tetrahedral bond angle
Calculating bond angles of a symmetrical tetrahedral molecule using a dot product

The bond angle for a symmetric tetrahedral molecule such as CH4 may be calculated using the dot product of two vectors. As shown in the diagram, the molecule can be inscribed in a cube with the tetravalent atom (e.g. carbon) at the cube centre which is the origin of coordinates, O. The four monovalent atoms (e.g. hydrogens) are at four corners of the cube (A, B, C, D) chosen so that no two atoms are at adjacent corners linked by only one cube edge. If the edge length of the cube is chosen as 2 units, then the two bonds OA and OB correspond to the vectors a = (1, –1, 1) and b = (1, 1, –1), and the bond angle θ is the angle between these two vectors. This angle may be calculated from the dot product of the two vectors, defined as a • b = ||a|| ||b|| cos θ where ||a|| denotes the length of vector a. As shown in the diagram, the dot product here is –1 and the length of each vector is √3, so that cos θ = –1/3 and the tetrahedral bond angle θ = arccos(–1/3) ≃ 109.47°.

Examples

Main group chemistry
The tetrahedral molecule methane (CH4)

Aside from virtually all saturated organic compounds, most compounds of Si, Ge, and Sn are tetrahedral. Often tetrahedral molecules feature multiple bonding to the outer ligands, as in xenon tetroxide (XeO4), the perchlorate ion (ClO−4), the sulfate ion (SO2−4), the phosphate ion (PO3−4). Thiazyl trifluoride (SNF3) is tetrahedral, featuring a sulfur-to-nitrogen triple bond.[3]

Other molecules have a tetrahedral arrangement of electron pairs around a central atom; for example ammonia (NH3) with the nitrogen atom surrounded by three hydrogens and one lone pair. However the usual classification considers only the bonded atoms and not the lone pair, so that ammonia is actually considered as pyramidal. The H–N–H angles are 107°, contracted from 109.5°. This difference is attributed to the influence of the lone pair which exerts a greater repulsive influence than a bonded atom.

Transition metal chemistry

Again the geometry is widespread, particularly so for complexes where the metal has d0 or d10 configuration. Illustrative examples include tetrakis(triphenylphosphine)palladium(0) (Pd[P(C6H5)3]4), nickel carbonyl (Ni(CO)4), and titanium tetrachloride (TiCl4). Many complexes with incompletely filled d-shells are often tetrahedral, e.g. the tetrahalides of iron(II), cobalt(II), and nickel(II).

Water structure

In the gas phase, a single water molecule has an oxygen atom surrounded by two hydrogens and two lone pairs, and the H2O geometry is simply described as bent without considering the nonbonding lone pairs.

However, in liquid water or in ice, the lone pairs form hydrogen bonds with neighboring water molecules. The most common arrangement of hydrogen atoms around an oxygen is tetrahedral with two hydrogen atoms covalently bonded to oxygen and two attached by hydrogen bonds. Since the hydrogen bonds vary in length many of these water molecules are not symmetrical and form transient irregular tetrahedra between their four associated hydrogen atoms.[4]

Bitetrahedral structures

Many compounds and complexes adopt bitetrahedral structures. In this motif, the two tetrahedra share a common edge. The inorganic polymer silicon disulfide features an infinite chain of edge-shared tetrahedra. In a completely saturated hydrocarbon system, bitetrahedral molecule C8H6 has been proposed as a candidate for the molecule with the shortest possible carbon-carbon single bond.[5]

Bitetrahedral structure adopted by Al2Br6 ("aluminium tribromide") and Ga2Cl6 ("gallium trichloride").

Exceptions and distortions

Inversion of tetrahedra occurs widely in organic and main group chemistry. The Walden inversion illustrates the stereochemical consequences of inversion at carbon. Nitrogen inversion in ammonia also entails transient formation of planar NH3.

Inverted tetrahedral geometry

Geometrical constraints in a molecule can cause a severe distortion of idealized tetrahedral geometry. In compounds featuring "inverted" tetrahedral geometry at a carbon atom, all four groups attached to this carbon are on one side of a plane.[6] The carbon atom lies at or near the apex of a square pyramid with the other four groups at the corners.[7][8]

Inverted carbon

The simplest examples of organic molecules displaying inverted tetrahedral geometry are the smallest propellanes, such as [1.1.1]propellane; or more generally the paddlanes,[9] and pyramidane ([3.3.3.3]fenestrane).[7][8] Such molecules are typically strained, resulting in increased reactivity.

Planarization

A tetrahedron can also be distorted by increasing the angle between two of the bonds. In the extreme case, flattening results. For carbon this phenomenon can be observed in a class of compounds called the fenestranes.

Tetrahedral molecules with no central atom

A few molecules have a tetrahedral geometry with no central atom. An inorganic example is tetraphosphorus (P4) which has four phosphorus atoms at the vertices of a tetrahedron and each bonded to the other three. An organic example is tetrahedrane (C4H4) with four carbon atoms each bonded to one hydrogen and the other three carbons. In this case the theoretical C−C−C bond angle is just 60° (in practice the angle will be larger due to bent bonds), representing a large degree of strain.

See also

References
  1. Alger, Nick. "Angle Between 2 Legs of a Tetrahedron". Archived from the original on 2018-10-03.
  2. Brittin, W. E. (1945). "Valence Angle of the Tetrahedral Carbon Atom". J. Chem. Educ. 22 (3): 145. Bibcode:1945JChEd..22..145B. doi:10.1021/ed022p145.
  3. Miessler, G. L.; Tarr, D. A. (2004). Inorganic Chemistry (3rd ed.). Pearson/Prentice Hall. ISBN 0-13-035471-6.
  4. Mason, P. E.; Brady, J. W. (2007). ""Tetrahedrality" and the Relationship between Collective Structure and Radial Distribution Functions in Liquid Water". J. Phys. Chem. B. 111 (20): 5669–5679. doi:10.1021/jp068581n. PMID 17469865.
  5. Xie, Yaoming; Schaefer, Henry F. (1989-09-29). "The bitetrahedral molecule C8H6: The shortest possible CC bond distance for a saturated hydrocarbon?". Chemical Physics Letters. 161 (6): 516–518. Bibcode:1989CPL...161..516X. doi:10.1016/0009-2614(89)87031-9. ISSN 0009-2614.
  6. Wiberg, Kenneth B. (1984). "Inverted geometries at carbon". Acc. Chem. Res. 17 (11): 379–386. doi:10.1021/ar00107a001.
  7. Joseph P. Kenny; Karl M. Krueger; Jonathan C. Rienstra-Kiracofe; Henry F. Schaefer III (2001). "C5H4: Pyramidane and Its Low-Lying Isomers". J. Phys. Chem. A. 105 (32): 7745–7750. Bibcode:2001JPCA..105.7745K. doi:10.1021/jp011642r.
  8. Lewars, E. (1998). "Pyramidane: an ab initio study of the C5H4 potential energy surface". Journal of Molecular Structure: THEOCHEM. 423 (3): 173–188. doi:10.1016/S0166-1280(97)00118-8.
  9. IUPAC, Compendium of Chemical Terminology, 2nd ed. (the "Gold Book") (1997). Online corrected version: (2006–) "paddlanes". doi:10.1351/goldbook.P04395
This article is issued from Wikipedia. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.