partial order
See also: partial-order
English
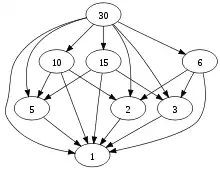
Hasse diagram of a set with the partial order "is divisible by"
Noun
partial order (plural partial orders)
- (set theory, order theory) A binary relation that is reflexive, antisymmetric, and transitive.
- 1986, Kenneth R. Goodearl, Partially Ordered Abelian Groups with Interpolation, American Mathematical Society, Softcover reprint 2010, page xxi,
- A partial order on a set X is any reflexive, antisymmetric, transitive relation on X. In most cases, partial orders are denoted ≤.
- 1999, Paul A. S. Ward, An Online Algorithm for Dimension-Bound Analysis, Patrick Amestoy, P. Berger, M. Daydé, I. Duff, V. Frayssé, L. Giraud, D. Ruiz (editors), Euro-Par ’99 Parallel Processing: 5th International Euro-Par Conference, Proceedings, Springer, LNCS 1685, page 144,
- The vector-clock size necessary to characterize causality in a distributed computation is bounded by the dimension of the partial order induced by that computation.
- 2008, David Eppstein, Jean-Claude Falmagne, Sergei Ovchinnikov, Media Theory: Interdisciplinary Applied Mathematics, Springer, page 7,
- Consider an arbitrary finite set S. The family of all strict partial orders (asymmetric, transitive, cf. 1.8.3, p. 14) on S enjoys a remarkable property: any partial order P can be linked to any other partial order P’ by a sequence of steps each of which consists of changing the order either by adding one ordered pair of elements of S (imposing an ordering between two previously-incomparable elements) or by removing one ordered pair (causing two previously related elements to become incomparable), without ever leaving the family .
- 1986, Kenneth R. Goodearl, Partially Ordered Abelian Groups with Interpolation, American Mathematical Society, Softcover reprint 2010, page xxi,
Synonyms
Hypernyms
Hyponyms
Related terms
Translations
binary relation that is reflexive, antisymmetric, and transitive
|
|
Further reading


References
- B. Dushnik and E. W. Miller, Partially Ordered Sets, Amer. J. Math. 63 (1941), 600-610.
This article is issued from Wiktionary. The text is licensed under Creative Commons - Attribution - Sharealike. Additional terms may apply for the media files.