When the function to be integrated has a cylindrical symmetry, it is sensible to change the variables into cylindrical coordinates and then perform integration.
In R3 the integration on domains with a circular base can be made by the passage in cylindrical coordinates; the transformation of the function is made by the following relation:
The domain transformation can be graphically attained, because only the shape of the base varies, while the height follows the shape of the starting region. Also in switching to cylindrical coordinates, the
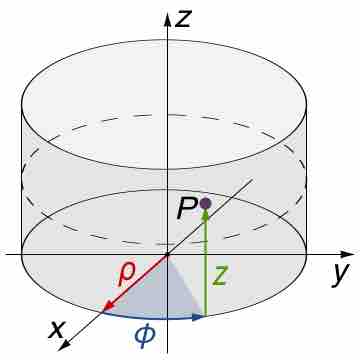
Cylindrical Coordinates
Cylindrical coordinates are often used for integrations on domains with a circular base.
Example 1
The region is:
If the transformation is applied, this region is obtained:
because the z component is unvaried during the transformation, the
This method is convenient in case of cylindrical or conical domains or in regions where it is easy to individuate the
Example 2
The function
The transformation of
while the function becomes:
Therefore, the integral becomes: