The Substitution Rule

Integration by substitution, also known as $u$-substitution, is a method for finding integrals. Using the fundamental theorem of calculus often requires finding an antiderivative. For this and other reasons, integration by substitution is an important tool for mathematicians. It is the counterpart to the chain rule of differentiation.

Definite Integral

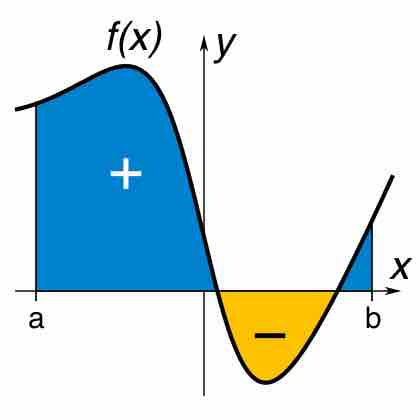
A definite integral of a function can be represented as the signed area of the region bounded by its graph.

Let $I\subseteq \mathbb{R}$ be an interval and $g:[a, b] \rightarrow 1$ be a continuously differentiable function. Suppose that $f:I \rightarrow \mathbb{R}$ is a continuous function. Then:

$\displaystyle{\int_{g(a)}^{g(b)}f(x)dx = \int_{a}^{b}f(g(t))g'(t)dt}$

Using Leibniz notation, the substitution $x = g(t)$ yields:

$\displaystyle{\frac{dx}{dt} = g'(t)}$

and therefore, formally:

$dx = g'(t)dt$

which is the required substitution for $dx$.

The formula is used to transform one integral into another integral that is easier to compute. Thus, the formula can be used from left to right or from right to left in order to simplify a given integral. When used in the latter manner, it is often known as $u$-substitution (or $w$-substitution).

For example, consider the following integral:

$\displaystyle{\int_0^2 x \cos(x^2+1) dx}$

If we make the substitution $u = x^2 + 1$, we obtain $du = 2x dx$  and

$\begin{aligned} \displaystyle{\int_{x=0}^{x=2}x \cos(x^{2}+1)} &= \displaystyle{ \frac{1}{2}\int_{u=1}^{u=5} \cos(u)du} \\ &= \frac{1}{2} \left (\sin(5) - \sin(1) \right ) \end{aligned}$

It is important to note that since the lower limit $x = 0$ was replaced with $u = 0^2 + 1 = 1$, and the upper limit $x=2$  replaced with $u = 2^2 + 1 = 5$ , a transformation back into terms of $x$ was unnecessary.

Substitution can be used to determine antiderivatives if one chooses a relation between $x$ and $u$, determines the corresponding relation between $dx$ and $du$ by differentiating, and performs the substitutions. An antiderivative for the substituted function can hopefully be determined; the original substitution between $u$ and $x$ is then undone.

Similar to our first example above, we can determine the following antiderivative with this method: 

$\displaystyle{\int x \cos(x^{2}+1)}$

$\displaystyle{= \frac{1}{2}\int 2x\cos(x^{2}+1)dx}$

$\displaystyle{= \frac{1}{2}\int \cos(u)du}$

$\displaystyle{ = \frac{1}{2}\sin(u) + C}$

$\displaystyle{ = \frac{1}{2} \sin(x^{2}+1)+C}$

where $C$ is an arbitrary constant of integration. Note that there were no integral boundaries to transform, but in the last step we had to revert the original substitution, $u = x^2 + 1$.