Taylor Polynomials

Taylor series is a representation of a function as an infinite sum of terms that are calculated from the values of the function's derivatives at a single point. The Taylor series of a real or complex-valued function $f(x)$ that is infinitely differentiable in a neighborhood of a real or complex number $a$ is the power series 

$\displaystyle{f(x) = \sum_{n=0} ^ {\infty} \frac {f^{(n)}(a)}{n! } \, (x-a)^{n}}$

where $n!$ denotes the factorial of $n$ and $f^{(n)}(a)$ denotes the $n$th derivative of $f$ evaluated at the point $x=a$. Any finite number of initial terms of the Taylor series of a function is called a Taylor polynomial.

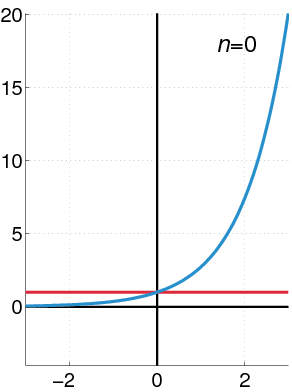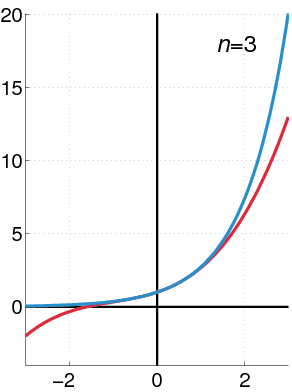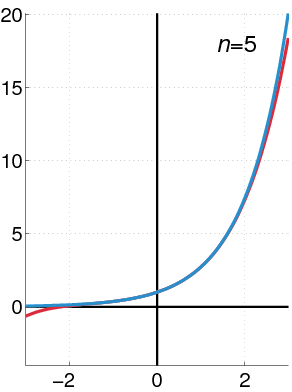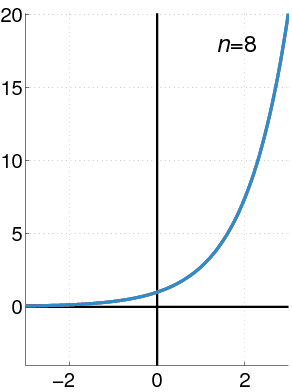
Exponential Function as a Taylor Series

The exponential function (in blue) and the sum of the first 9 terms of its Taylor series at 0 (in red).

Example

The Taylor series for the exponential function $e^x$ at $a=0$ is:

$\displaystyle{e^x = \sum_{n=0}^{\infty} \frac{x^n}{n! } = 1 + \frac{x^1}{1! } + \frac{x^2}{2! } + \frac{x^3}{3! } + \cdots}$

Using Taylor Series to Evaluate an Integral

Taylor series can be used to evaluate an integral when there is no other integration technique available (of course, other than numerical integration). Let's assume that the integration of a function ($f(x)$) cannot be performed analytically. To evaluate the integral $I = \int_{a}^{b} f(x) \, dx$, we can Taylor-expand $f(x)$ and perform integration on individual terms of the series. Since $f(x) = \sum_{n=0} ^ {\infty} \frac {f^{(n)}(0)}{n! } \, x^{n}$, we get:

$\displaystyle{I = \sum_{n=0} ^ {\infty} \frac {f^{(n)}(0)}{n! } \, \int_{a}^{b}x^{n}\, dx \\ \, \,= \sum_{n=0} ^ {\infty} \frac {f^{(n)}(0)}{(n+1)! } \, (b^{n+1}-a^{n+1})}$

Therefore, as long as Taylor expansion is possible and the infinite sum converges, the definite integral ($I$) can be evaluated.