Kepler's First Law
Kepler's first law states that
The orbit of every planet is an ellipse with the Sun at one of the two foci.
An ellipse is a closed plane curve that resembles a stretched out circle. Note that the Sun is not at the center of the ellipse, but at one of its foci. The other focal point,
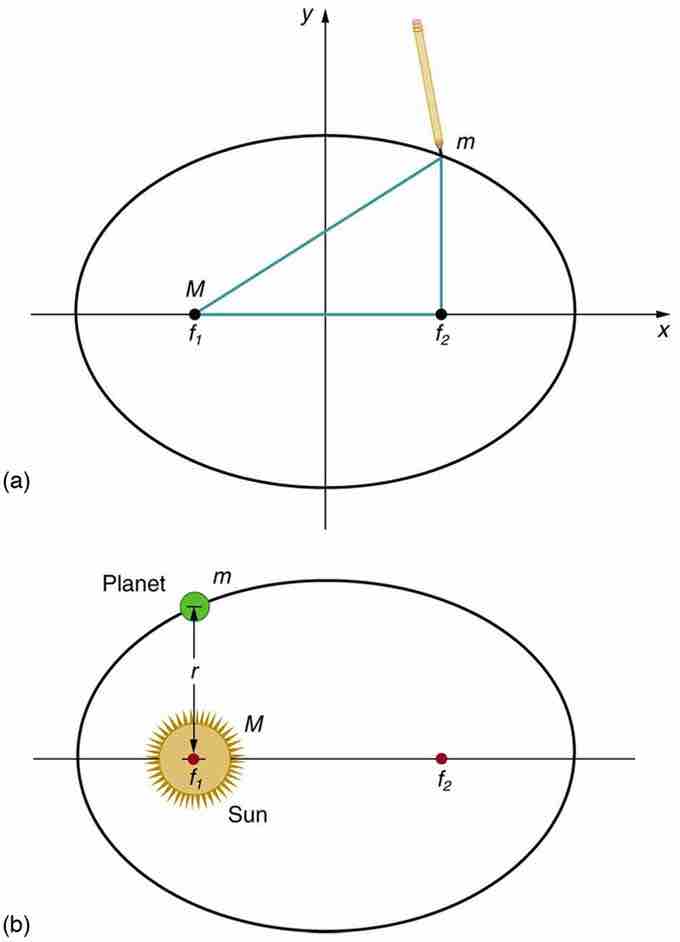
Ellipses and Kepler's First Law
(a) An ellipse is a closed curve such that the sum of the distances from a point on the curve to the two foci (
How stretched out an ellipse is from a perfect circle is known as its eccentricity: a parameter that can take any value greater than or equal to 0 (a circle) and less than 1 (as the eccentricity tends to 1, the ellipse tends to a parabola). The eccentricities of the planets known to Kepler varied from 0.007 (Venus) to 0.2 (Mercury). Minor bodies such as comets an asteroids (discovered after Kepler's time) can have very large eccentricities. The dwarf planet Pluto, discovered in 1929, has an eccentricity of 0.25.
Symbolically, an ellipse can be represented in polar coordinates as:
where

Orbit As Ellipse
Heliocentric coordinate system
At
At
At
The semi-major axis
The semi-minor axis
The semi-latus rectum
The eccentricity
The area of the ellipse is
The special case of a circle is