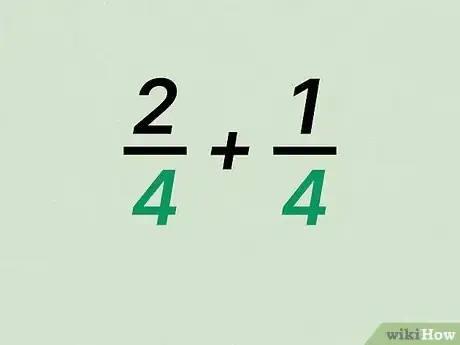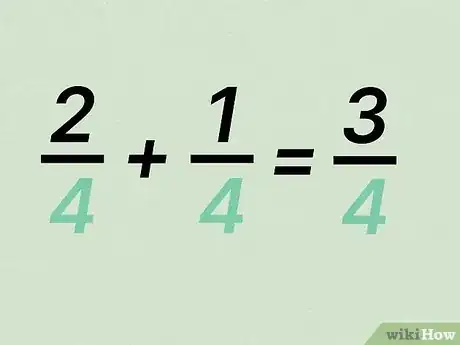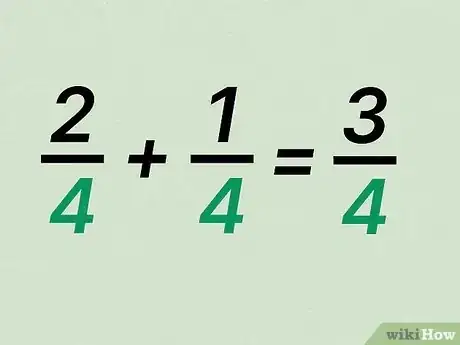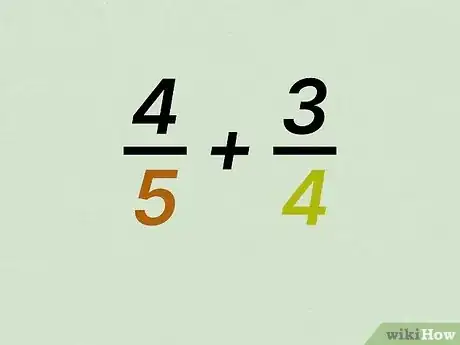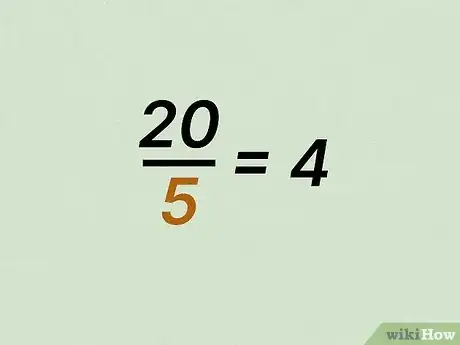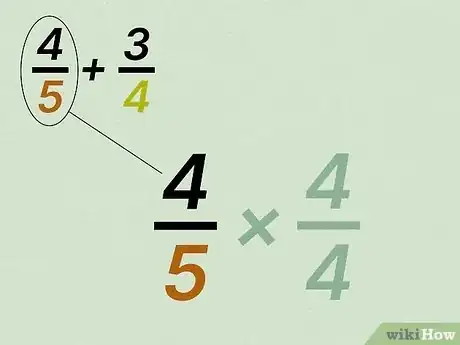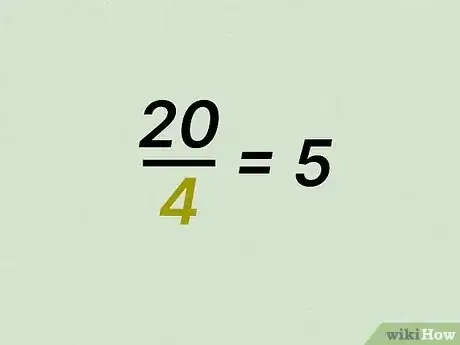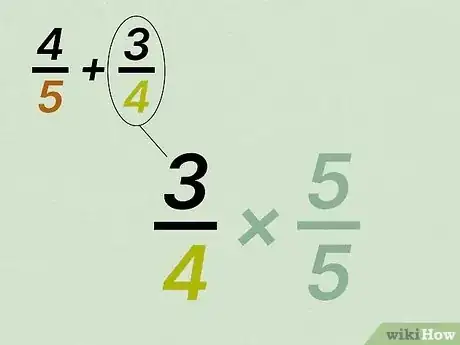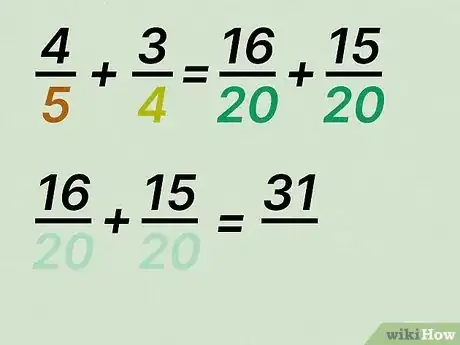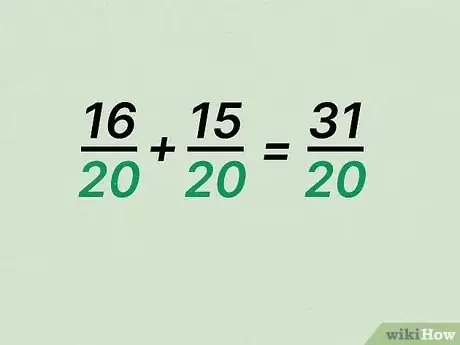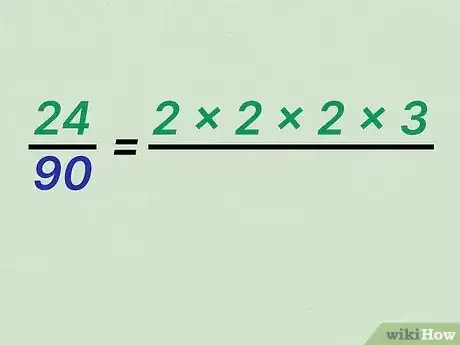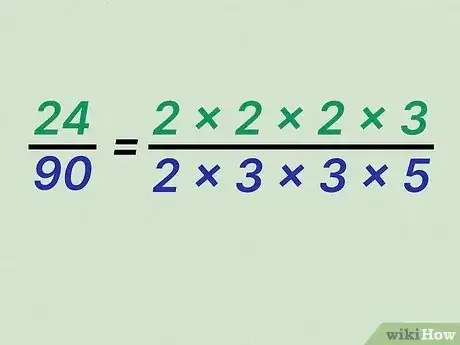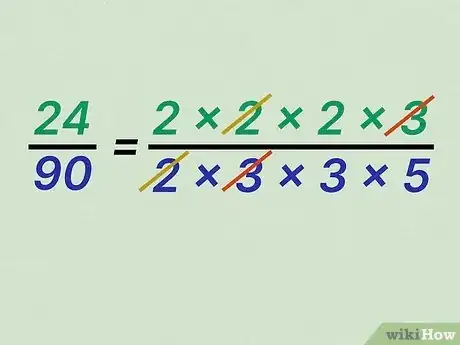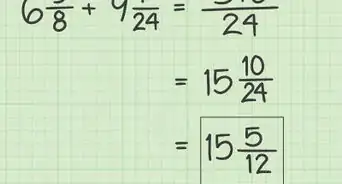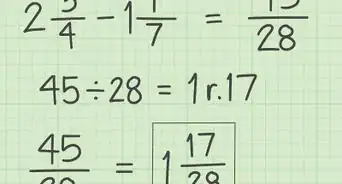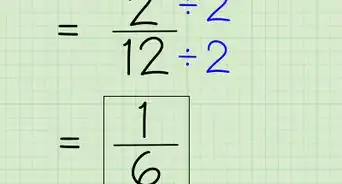This article was co-authored by David Jia. David Jia is an Academic Tutor and the Founder of LA Math Tutoring, a private tutoring company based in Los Angeles, California. With over 10 years of teaching experience, David works with students of all ages and grades in various subjects, as well as college admissions counseling and test preparation for the SAT, ACT, ISEE, and more. After attaining a perfect 800 math score and a 690 English score on the SAT, David was awarded the Dickinson Scholarship from the University of Miami, where he graduated with a Bachelor’s degree in Business Administration. Additionally, David has worked as an instructor for online videos for textbook companies such as Larson Texts, Big Ideas Learning, and Big Ideas Math.
There are 11 references cited in this article, which can be found at the bottom of the page.
This article has been viewed 52,208 times.
Once you understand the concept of fractions, you can start performing simple operations with them. You can add fractions just like you can add other types of numbers. The important thing to remember, though, is that fractions must have the same denominator before you can add them. Once you find the sum of two fractions, you will likely need to simplify it, or reduce it.
Steps
Adding Fractions with Like Denominators
-
1Verify the fractions have the same denominator. A denominator is the number below the fraction bar.[1] If the fractions do not have the same denominator, you cannot use this method.
- For example, if you are calculating , you can note that both fractions have the same denominator: 4.
-
2Add the numerators. A numerator is the number above the fraction bar. Add numerators the same way you would add integers.[2]
- For example, the numerators of and are 2 and 1, so you would calculate . So, 3 is the numerator of your sum.
Advertisement -
3Place the numerators’ sum over the denominator. Since both fractions you are adding have the same denominator, the denominator of their sum will also be the same.[3]
- For example, the sum of will have a denominator of 4: .
Adding Fractions with Unlike Denominators
-
1Verify the fractions have different denominators. A denominator is the number below the fraction bar.[4]
- For example, if you are calculating , you can note that the fractions have different denominators: 5 and 4.
-
2List the first several multiples of the smaller denominator. A multiple is a number that another number equally divides into. You can also think of a multiple as the result of multiplying a number by a whole number. You are looking for the smallest multiple that the two denominators have in common.[5]
- For example, the smallest denominator in is 4. The first several multiples of 4 are 4, 8, 12, 16, and 20. The smallest of these multiples that 5 shares with 4 is 20. So, 20 is the least common multiple of the two denominators.
-
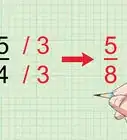
3Divide the first fraction’s denominator into the least common multiple. The result will give you a factor of change. This factor tells you how much bigger the common multiple is than the denominator.[6]
- For example, if the least common multiple is 20, and the first fraction’s denominator is 5, you would calculate . That means 4 is the factor of change. The least common multiple is 4 times larger than the denominator.
-
4
-
5Write the first fraction’s equivalent fraction. The numerator will be the product of the factor of change and the original fraction’s numerator. The denominator will be the least common multiple.
- For example, .
-
6Divide the second fraction’s denominator into the least common multiple. The result will give you a factor of change for the second fraction. This factor tells you how much bigger the common multiple is than the denominator.
- For example, if the least common multiple is 20, and the second fraction’s denominator is 4, you would calculate . That means 5 is the factor of change for the second fraction.
-
7Multiply the numerator of the second fraction by the factor of change. This will give you the numerator of your equivalent fraction.[9]
- For example, if the factor of change is 5, and the second fraction’s numerator is 3, you would calculate .
-
8Write the second fraction’s equivalent fraction. The numerator will be the product of the factor of change and the original fraction’s numerator. The denominator will be the least common multiple.[10]
- For example, .
-
9Add the numerators of the equivalent fractions. Since the equivalent fractions have the same denominator, you can add the numerators as you normally would.[11]
- For example, .
-
10Place the sum of the numerators over the new denominator. Make sure you use the common denominator of the equivalent fractions.[12]
- For example, .
Simplifying Fractions
-
1Factor the numerator. You want to factor the numerator into all of its prime factors. Remember that a prime number is a number that is only divisible by 1 and itself. Rewrite the fraction showing this prime factorization in the numerator.[13]
- For example, if simplifying the fraction , you would calculate that . So, rewrite the fraction as
-
2Factor the denominator. You also want to factor the denominator into its prime factors. Rewrite the fraction showing its prime factorization in the denominator.[14]
- For example, if simplifying the fraction , you would calculate that . So, rewrite the fraction as .
-
3Cancel the factors common to the numerator and denominator. Remember that when a factor is common to the top and bottom of a fraction, it cancels to . This means you can eliminate these factors, since any number multiplied by 1 is itself.[15]
- For example, you can cancel out a 2 and a 3 in the numerator and denominator: .
-
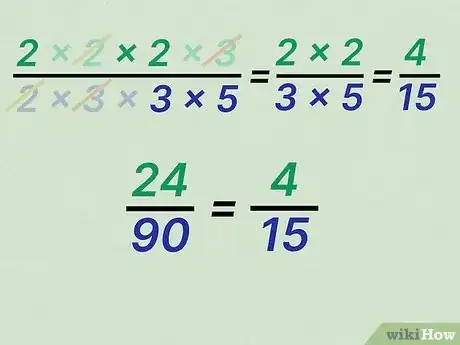
4Rewrite the fraction with the remaining factors. You want to simplify the fraction so that it only includes the factors that did not cancel. If more than one factor remains in either the numerator or denominator, you need to multiply them together to get a single integer. The result will be your simplified fraction.[16]
- For example:
So, the fraction simplifies to .
- For example:
Community Q&A
-
QuestionHow do I simplify mixed numbers in BEDMAS form, and how can I simplify fractions the fastest way?
 DonaganTop AnswererBEDMAS is an order of arithmetic operations and has nothing to do with simplifying mixed numbers. The fastest way to simplify a fraction is to recognize the largest whole number that will divide evenly into both the numerator and denominator. For example, with the fraction 30/45, the largest integer (whole number) that will divide evenly into both 30 and 45 is 15, which divides into the numerator two times and the denominator three times. So 30/45 simplified is 2/3.
DonaganTop AnswererBEDMAS is an order of arithmetic operations and has nothing to do with simplifying mixed numbers. The fastest way to simplify a fraction is to recognize the largest whole number that will divide evenly into both the numerator and denominator. For example, with the fraction 30/45, the largest integer (whole number) that will divide evenly into both 30 and 45 is 15, which divides into the numerator two times and the denominator three times. So 30/45 simplified is 2/3. -
QuestionHow do you simplify?
 Zoe BullockCommunity AnswerLook at the number and think, how many times can this number go into this number? Then you divide. Example: 20/60, 20 can go into 60 three times, so your answer is 3 wholes.
Zoe BullockCommunity AnswerLook at the number and think, how many times can this number go into this number? Then you divide. Example: 20/60, 20 can go into 60 three times, so your answer is 3 wholes.
References
- ↑ https://www.mathsisfun.com/fractions_addition.html
- ↑ https://www.khanacademy.org/math/arithmetic/fraction-arithmetic/arith-review-add-sub-fractions/v/adding-small-fractions-with-unlike-denominators
- ↑ http://www.helpwithfractions.com/adding-fractions-same-denominator/
- ↑ https://www.mathsisfun.com/fractions_addition.html
- ↑ https://www.khanacademy.org/math/arithmetic/fraction-arithmetic/arith-review-add-sub-fractions/v/adding-small-fractions-with-unlike-denominators
- ↑ https://virtualnerd.com/pre-algebra/rational-numbers/fraction-addition-subtraction/add-fractions/add-fractions-different-denominator
- ↑ David Jia. Academic Tutor. Expert Interview. 7 January 2021.
- ↑ http://www.coolmath.com/prealgebra/01-fractions/fractions-12-adding-subtracting-different-denominators-01/
- ↑ https://virtualnerd.com/pre-algebra/rational-numbers/fraction-addition-subtraction/add-fractions/add-fractions-different-denominator
- ↑ https://virtualnerd.com/pre-algebra/rational-numbers/fraction-addition-subtraction/add-fractions/add-fractions-different-denominator
- ↑ https://www.mathsisfun.com/fractions_addition.html
- ↑ http://www.helpwithfractions.com/adding-fractions-different-denominators/
- ↑ https://www.chilimath.com/lessons/introductory-algebra/simplifying-fractions/
- ↑ http://www.helpwithfractions.com/math-homework-helper/reducing-fractions/
- ↑ http://www.wtamu.edu/academic/anns/mps/math/mathlab/beg_algebra/beg_alg_tut3_fractions.htm
- ↑ https://www.calculatorsoup.com/calculators/math/fractionssimplify.php
About This Article
To add fractions with different denominators, start by finding the least common multiple. Then, divide each fraction's denominator by that number and multiply the numerators by the numbers you get. Finally, add the new numerators together and use the least common multiple as the denominator. To simplify fractions, just factor the numerator and denominator, cancel the common factors, and rewrite the fraction without them. To learn how to add fractions with the same denominator, read on!