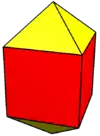
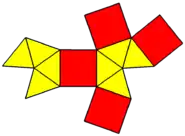
Elongated square bipyramid
In geometry, the elongated square bipyramid (or elongated octahedron) is one of the Johnson solids (J15). As the name suggests, it can be constructed by elongating an octahedron by inserting a cube between its congruent halves.
| Elongated square bipyramid | |
|---|---|
 | |
| Type | Johnson J14 – J15 – J16 |
| Faces | 8 triangles 4 squares |
| Edges | 20 |
| Vertices | 10 |
| Vertex configuration | 2(34) 8(32.42) |
| Symmetry group | D4h, [4,2], (*422) |
| Rotation group | D4, [4,2]+, (422) |
| Dual polyhedron | Square bifrustum |
| Properties | convex |
| Net | |
 | |

It has been named the pencil cube or 12-faced pencil cube due to its shape.[1][2]
A Johnson solid is one of 92 strictly convex polyhedra that is composed of regular polygon faces but are not uniform polyhedra (that is, they are not Platonic solids, Archimedean solids, prisms, or antiprisms). They were named by Norman Johnson, who first listed these polyhedra in 1966.[3]
A zircon crystal is an example of an elongated square bipyramid.
Formulae
The following formulae for volume (), surface area () and height () can be used if all faces are regular, with edge length :[4]
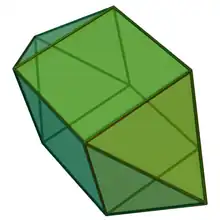
Dual polyhedron
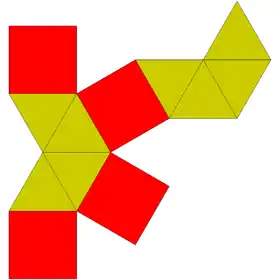
The dual of the elongated square bipyramid is called a square bifrustum and has 10 faces: 8 trapezoidal and 2 square.
| Dual elongated square bipyramid | Net of dual |
|---|---|
 |
 |
Related polyhedra and honeycombs
A special kind of elongated square bipyramid without all regular faces allows a self-tessellation of Euclidean space. The triangles of this elongated square bipyramid are not regular; they have edges in the ratio 2:√3:√3.
It can be considered a transitional phase between the cubic and rhombic dodecahedral honeycombs.[1] The cells are here colored white, red, and blue based on their orientation in space. The square pyramid caps have shortened isosceles triangle faces, with six of these pyramids meeting together to form a cube. The dual of this honeycomb is composed of two kinds of octahedra (regular octahedra and triangular antiprisms), formed by superimposing octahedra into the cuboctahedra of the rectified cubic honeycomb. Both honeycombs have a symmetry of [[4,3,4]].
Cross-sections of the honeycomb, through cell centers produces a chamfered square tiling, with flattened horizontal and vertical hexagons, and squares on the perpendicular polyhedra.
 Honeycomb |
 Half honeycomb |
 Chamfered square tiling |
With regular faces, the elongated square bipyramid can form a tessellation of space with tetrahedra and octahedra. (The octahedra can be further decomposed into square pyramids.)[5] This honeycomb can be considered an elongated version of the tetrahedral-octahedral honeycomb.
See also
References
- Order in Space: A design source book, Keith Critchlow, p.46-47
- Goldberg, Michael, On the space-filling octahedra, Geometriae Dedicata, January 1981, Volume 10, Issue 1, pp 323–335 PDF Archived 2017-12-22 at the Wayback Machine
- Johnson, Norman W. (1966), "Convex polyhedra with regular faces", Canadian Journal of Mathematics, 18: 169–200, doi:10.4153/cjm-1966-021-8, MR 0185507, Zbl 0132.14603.
- Sapiña, R. "Area and volume of the Johnson solid J15". Problemas y Ecuaciones (in Spanish). ISSN 2659-9899. Retrieved 2020-09-09.
- "J15 honeycomb".