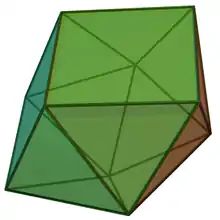
Sphenocorona
In geometry, the sphenocorona is one of the Johnson solids (J86). It is one of the elementary Johnson solids that do not arise from "cut and paste" manipulations of the Platonic and Archimedean solids.
| Sphenocorona | |
|---|---|
 | |
| Type | Johnson J85 – J86 – J87 |
| Faces | 2x2+2x4 triangles 2 squares |
| Edges | 22 |
| Vertices | 10 |
| Vertex configuration | 4(33.4) 2(32.42) 2x2(35) |
| Symmetry group | C2v |
| Dual polyhedron | - |
| Properties | convex |
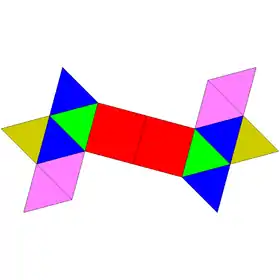
| Net | |
 | |

A Johnson solid is one of 92 strictly convex polyhedra that is composed of regular polygon faces but are not uniform polyhedra (that is, they are not Platonic solids, Archimedean solids, prisms, or antiprisms). They were named by Norman Johnson, who first listed these polyhedra in 1966.[1]
Johnson uses the prefix spheno- to refer to a wedge-like complex formed by two adjacent lunes, a lune being a square with equilateral triangles attached on opposite sides. Likewise, the suffix -corona refers to a crownlike complex of 8 equilateral triangles. Joining both complexes together results in the sphenocorona.[2]
Cartesian coordinates
Let k ≈ 0.85273 be the smallest positive root of the quartic polynomial
Then, Cartesian coordinates of a sphenocorona with edge length 2 are given by the union of the orbits of the points
under the action of the group generated by reflections about the xz-plane and the yz-plane.[3]
One may then calculate the surface area of a sphenocorona of edge length a as
and its volume as
Variations
The sphenocorona is also the vertex figure of the isogonal n-gonal double antiprismoid where n is an odd number greater than one, including the grand antiprism with pairs of trapezoid rather than square faces.
See also
References
- Johnson, Norman W. (1966), "Convex polyhedra with regular faces", Canadian Journal of Mathematics, 18: 169–200, doi:10.4153/cjm-1966-021-8, MR 0185507, Zbl 0132.14603.
- Johnson, Norman W. (1966), "Convex polyhedra with regular faces", Canadian Journal of Mathematics, 18: 169–200, doi:10.4153/cjm-1966-021-8, MR 0185507, S2CID 122006114, Zbl 0132.14603.
- Timofeenko, A. V. (2009). "The non-Platonic and non-Archimedean noncomposite polyhedra". Journal of Mathematical Science. 162 (5): 718. doi:10.1007/s10958-009-9655-0. S2CID 120114341.
- Wolfram Research, Inc. (2020). "Wolfram|Alpha Knowledgebase". Champaign, IL.
PolyhedronData[{"Johnson", 86}, "SurfaceArea"]{{cite journal}}: Cite journal requires|journal=(help) - Wolfram Research, Inc. (2020). "Wolfram|Alpha Knowledgebase". Champaign, IL.
PolyhedronData[{"Johnson", 86}, "Volume"]{{cite journal}}: Cite journal requires|journal=(help)
External links
- Weisstein, Eric W., "Sphenocorona" ("Johnson solid") at MathWorld.