X
wikiHow is a “wiki,” similar to Wikipedia, which means that many of our articles are co-written by multiple authors. To create this article, 29 people, some anonymous, worked to edit and improve it over time.
This article has been viewed 388,584 times.
Learn more...
Synthetic division is a shorthand method of dividing polynomials where you divide the coefficients of the polynomials, removing the variables and exponents. It allows you to add throughout the process instead of subtract, as you would do in traditional long division.[1] If you want to know how to divide polynomials using synthetic division, just follow these steps.
Steps
-
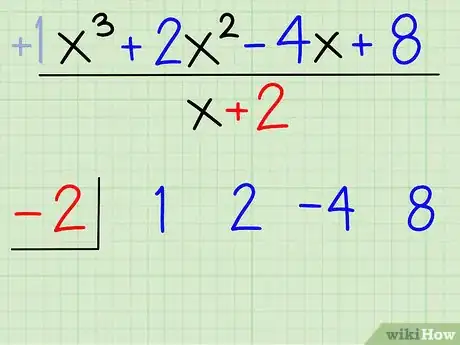
1Write down the problem. For this example, you will be dividing x3 + 2x2 - 4x + 8 by x + 2. Write the first polynomial equation, the dividend, in the numerator and write the second equation, the divisor, in the denominator.
-
2Reverse the sign of the constant in the divisor. The constant in the divisor, x + 2, is positive 2, so reversing the sign of the constant would give you -2.Advertisement
-
3Since the opposite of the constant term is -2, we put the -2 inside a box. A common mistake is to put a 2 inside the box, watch out here.
-
4Write all of the coefficients of the dividend inside the division symbol. [2] Write the terms from left to right, just as they appear. It should look like this: -2| 1 2 -4 8.
-
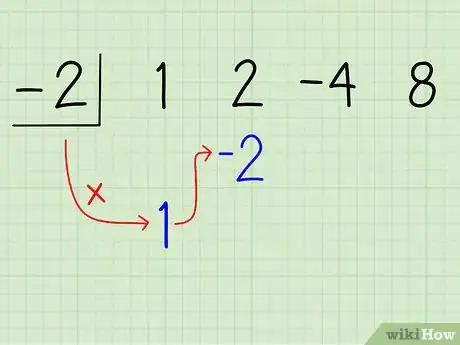
5Bring down the first coefficient. Bring down the first coefficient, 1, below itself. It should look like this:
-
-2| 1 2 -4 8
↓
1
-
-2| 1 2 -4 8
-
6Multiply the first coefficient by the divisor and place it under the second coefficient.[3] Simply multiply 1 by -2 to get -2 and write this product under the second term, 2. Here's how it would look:
-
-2| 1 2 -4 8
-2
1
-
-2| 1 2 -4 8
-
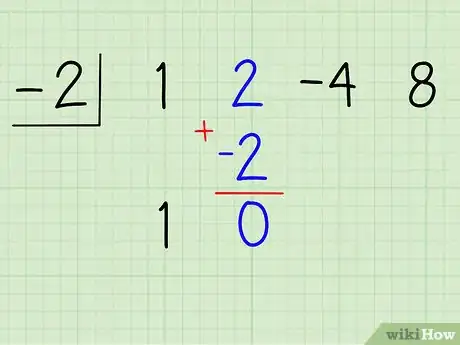
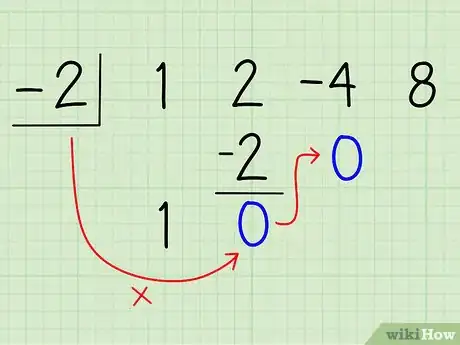
7Add the second coefficient and the product and write the answer below the product. Now take the second coefficient, 2, and add it to -2. The result is 0. Write this result below the two numbers, just as you would in long division. Here's how it would look:
-
-2| 1 2 -4 8
-2
1 0
-
-2| 1 2 -4 8
-
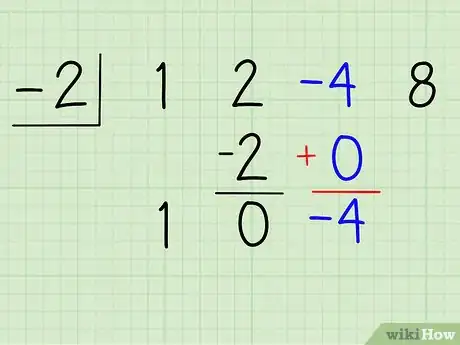
8Multiply this sum by the divisor and place the result under the third coefficient. Now take the sum, 0, and multiply it by the divisor, -2. The result is 0. Place this number below 4, the third coefficient. It should look like this:
-
-2| 1 2 -4 8
-2 0
1
-
-2| 1 2 -4 8
-
9Add the product and the third coefficient and write the result under the product. Add 0 and -4 to get -4 and write this answer below the 0. Here's how it would look:
-
-2| 1 2 -4 8
-2 0
1 0 -4
-
-2| 1 2 -4 8
-
10Multiply this number by the divisor, write it under the last coefficient, and add it to the coefficient. Now, multiply -4 by -2 to get 8, write this answer under the fourth coefficient, 8, and add this answer to the fourth coefficient. 8 + 8 = 16, so this is your remainder. Write this number below the product. Here's how it would look:
-
-2| 1 2 -4 8
-2 0 8
1 0 -4 |16
-
-2| 1 2 -4 8
-
11Place each of the new coefficients next to a variable of one less power than their original corresponding variables. In this case, the first sum, 1, is placed next to an x to the second power (one less than three). The second sum, 0, is placed next to an x, but the result is zero, so you can remove this term. And the third coefficient, -4, becomes a constant, a number without a variable, since the original variable was x. You can write an R next to the 16, because that is the remainder. Here's how it would look:
-
-2| 1 2 -4 8
-2 0 8
1 0 -4 |16
x2 + 0x - 4 R 16
x2 - 4 R16
-
-2| 1 2 -4 8
-
12Write the final answer. The final answer is the new polynomial, x2 - 4, plus the remainder, 16, over the original divisor, x + 2. Here's how it would look: x2 - 4 +16/(x +2).
Advertisement
Community Q&A
-
QuestionHow do I solve synthetic division if the divisor is more than three terms?
 Community AnswerThe divisor cannot be more than three terms. In synthetic division, the divisor is always in the form x-a.
Community AnswerThe divisor cannot be more than three terms. In synthetic division, the divisor is always in the form x-a. -
QuestionWhat is the benefit of synthetic division?
 Community AnswerYou can do it on a smaller piece of paper. Seriously, that's it. Synthetic is computationally identical to long division, but instead of writing powers of x everywhere, it uses its layout to keep the exponents straight.
Community AnswerYou can do it on a smaller piece of paper. Seriously, that's it. Synthetic is computationally identical to long division, but instead of writing powers of x everywhere, it uses its layout to keep the exponents straight. -
QuestionHow do I solve a problem dividing a polynomial by 2x-1 in synthetic division?
 Community Answer2x-1 is actually the same as x-(1/2), so you can do synthetic division putting +(1/2) in the box.
Community Answer2x-1 is actually the same as x-(1/2), so you can do synthetic division putting +(1/2) in the box.
Advertisement
References
- ↑ https://www.purplemath.com/modules/synthdiv.htm
- ↑ https://www.purplemath.com/modules/synthdiv.htm
- ↑ http://www.mesacc.edu/~scotz47781/mat120/notes/divide_poly/synthetic/synthetic_division.html
- ↑ http://www.montereyinstitute.org/courses/DevelopmentalMath/COURSE_TEXT2_RESOURCE/U11_L2_T6_text_final.html
- PurpleMath.com - Great help with Algebra I and II
- Ruffini's Rule (Synthetic Division) on Wikipedia
About This Article
Advertisement





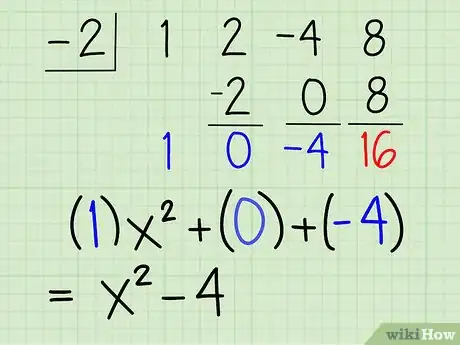
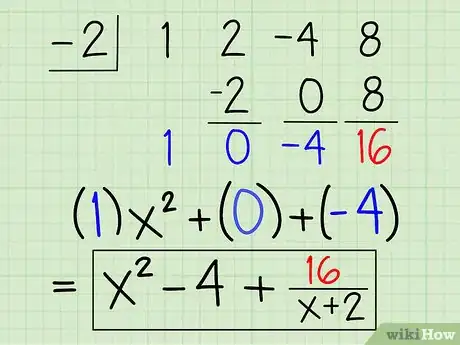

-Step-31-Version-2.webp)












-Step-31-Version-2.webp)