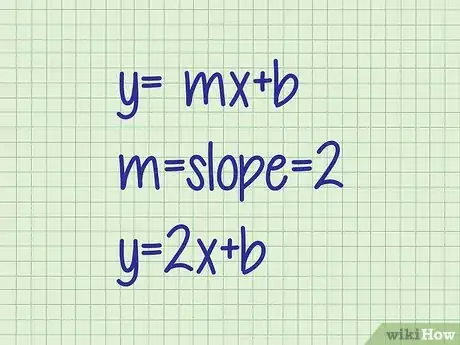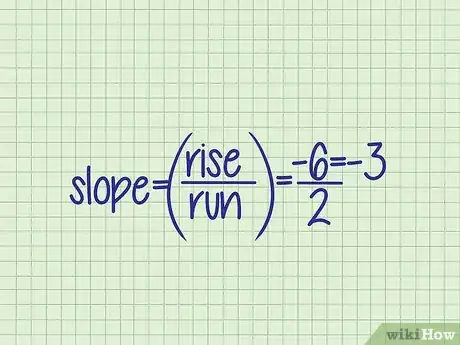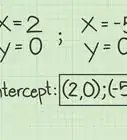How to Find the Y Intercept

Last Updated: February 3, 2023 References

On their own, y-intercepts aren’t complicated at all—they’re simply points where the graph of the equation intersects with the Y-axis.^{[1]
X
Research source} But how are you supposed to find a y-intercept when you only have a limited amount of info on hand? Thankfully, it’s not nearly as hard as it looks. We’ll walk you through all the major ways to find the y-intercept when you already have the slope and a point, two points, or just want to use a simple equation. With a little practice, you can make quick work of any y-intercept problem that comes your way.

Method 1

Method 1 of 3:

Finding the Y-Intercept from the Slope and Point

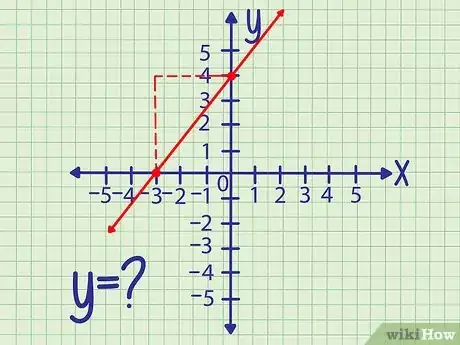
1
Write down the slope and point.^{[2]
X
Research source} The slope or "rise over run" is a single number that tells you how steep the line is. This type of problem also gives you the (x,y) coordinate of one point along the graph. Skip to the other methods below if you don't have both these pieces of information.
- Example 1: A straight line with slope 2 contains the point (-3,4). Find the y-intercept of this line using the steps below.
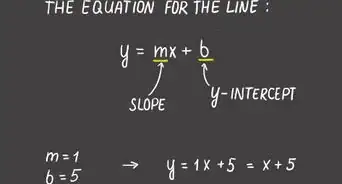
2
Learn the slope-intercept form of an equation. Any straight line can be written as an equation in the form y = mx + b. When the equation is in this form, the variable m is the slope, and b is the y-intercept.

Advertisement
3
Substitute the slope in this equation. Write the slope-intercept equation, but instead of m, use the slope of your line.
- Example 1 (cont.): y = mx + b
  m = slope = 2
  y = 2x + b
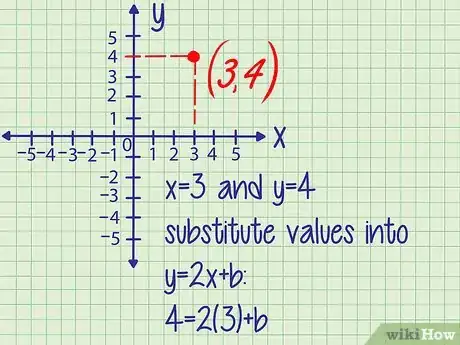
4
Replace x and y with the coordinates of the point. Any time you have the coordinates of a single point on your line, you can substitute those x and y coordinates for the x and y in your line equation. Do this for the equation you've been working on.
- Example 1 (cont.): The point (3,4) is on this line. At this point, x = 3 and y = 4.
  Substitute these values into y = 2x +b:
  4 = 2(3) + b
5
Solve for b. Remember, b is the y-intercept of the line. Now that b is the only variable in the equation, rearrange to solve for this variable and find the answer.
- Example 1 (cont.): 4 = 2(3) + b
  4 = 6 + b
  4 - 6 = b
  -2 = b
  The y-intercept of this line is -2.
6
Write this as a coordinate point. The y-intercept is the point where the line intersects with the y-axis. Since the y-axis is located at x = 0, the x coordinate of the y-intercept is always 0.
- Example 1 (cont.): The y-intercept is at y = -2, so the coordinate point is (0, -2).

Method 2

Method 2 of 3:

Using Two Points

1
Write down the coordinates of both points.^{[3]
X
Research source} This method covers problems that only tell you two points on a straight line.^{[4]
X
Research source} Write each point coordinate down in (x,y) form.
- Example 2: A straight line passes through points (-1, 2) and (3, -4). Find the y-intercept of this line using the steps below.
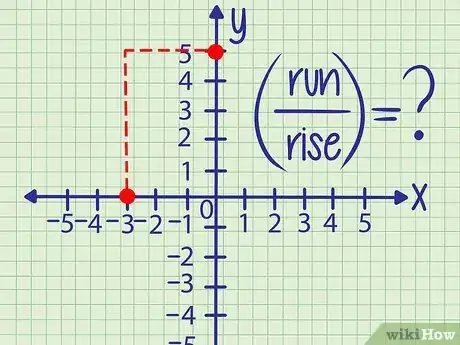
2
Calculate the rise and run. Slope is a measure of how much vertical distance the line moves for each unit of horizontal distance. You may have heard this described as "rise over run" ( ${\frac {rise}{run}}$ ${\frac {rise}{run}}$ ).^{[5]
X
Expert Source

Grace Imson, MA
Math Teacher
Expert Interview. 1 November 2019.} Here's how to find these two quantities from two points:
- "Rise" is the change in vertical distance, or the difference between the y-values of the two points.
- "Run" is the change in horizontal distance, or the difference between x-values of the same two points.
- Example 2 (cont.): The y-values of the two points are 2 and -4, so the rise is (-4) - (2) = -6.
  The x-values of the two points (in the same order) are 1 and 3, so the run is 3 - 1 = 2.
3
Divide rise by run to find the slope. Now that you know these two values, plug them into " ${\frac {rise}{run}}$ ${\frac {rise}{run}}$ " to find the slope of the line.
- Example 2 (cont.): $slope={\frac {rise}{run}}={\frac {-6}{2}}=$ -3.
4
Review the slope-intercept form. You can describe a straight line with the formula y = mx + b, where m is the slope and b is the y-intercept.^{[6]
X
Expert Source

Grace Imson, MA
Math Teacher
Expert Interview. 1 November 2019.} Now that we know the slope m and a point (x,y), we can use this equation to solve for b, the y-intercept.
5
Fit the slope and point into the equation. Take the equation in slope-intercept form and replace m with the slope you calculated. Replace the x and y terms with the coordinates of a single point on the line.^{[7]
X
Expert Source

Grace Imson, MA
Math Teacher
Expert Interview. 1 November 2019.} It doesn't matter which point you use.
- Example 2 (cont.): y = mx + b
  Slope = m = -3, so y = -3x + b
  The line includes a point with (x,y) coordinates (1,2), so 2 = -3(1) + b.
6
Solve for b. Now the only variable left in the equation is b, the y-intercept. Rearrange the equation so b is on one side, and you have your answer.^{[8]
X
Expert Source

Grace Imson, MA
Math Teacher
Expert Interview. 1 November 2019.} Remember, the y-intercept always has an x-coordinate of 0.
- Example 2 (cont.): 2 = -3(1) + b
  2 = -3 + b
  5 = b
  The y-intercept is at (0,5).

Method 3

Method 3 of 3:

Using an Equation

1
Write down the equation of the line. If you already have the equation of the line, you can find the y-intercept with a little algebra.^{[9]
X
Research source}
- Example 3: What is the y-intercept of the line x + 4y = 16?
- Note: Example 3 is a straight line. See the end of this section for an example of a quadratic equation (with a variable raised to the power of 2).
2
Substitute 0 for x. The y-axis is a vertical line along x = 0. This means any point on the y-axis has an x-coordinate of 0, including the y-intercept of the line. Plug in 0 for x in the line equation.
- Example 3 (cont.): x + 4y = 16
  x = 0
  0 + 4y = 16
  4y = 16
3
Solve for y. The answer is the y-intercept of the line.
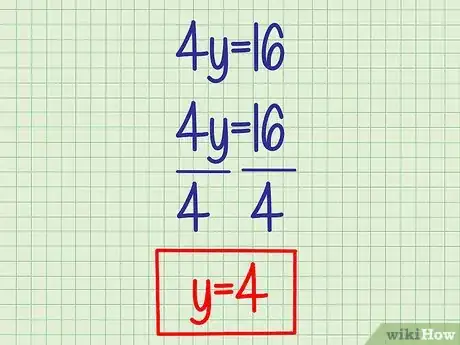
- Example 3 (cont.): 4y = 16
  ${\frac {4y}{4}}={\frac {16}{4}}$
  y = 4.
  The y-intercept of the line is 4.
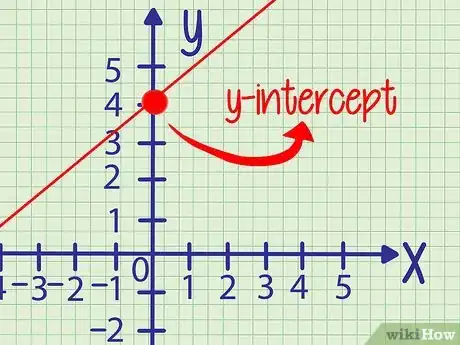
4
Confirm by graphing (optional). To check your answer, graph the equation as neatly as you can. The point where the line crosses the y-axis is the y-intercept.
5
Find the y-intercept for a quadratic equation. A quadratic equation includes a variable (x or y) raised to the power of 2. You can solve for y with the same substitution, but since the quadratic describes a curve, it could intercept the y-axis at 0, 1, or 2 points. This means you may end up with 0, 1, or 2 answers.
- Example 4: To find the y-intercept of $y^{2}=x+1$ , substitute x = 0 and solve the quadratic equation.
  In this case, we can solve $y^{2}=0+1$ by taking the square root of both sides. Remember, when taking a square root, you must account for two answers: a negative and a positive.
  ${\sqrt {y^{2}}}={\sqrt {1}}$
  y = 1 or y = -1. These are both y-intercepts of this curve.

Practice Problems and Answers

Practice Problems and Answers to Find the Y Intercept

Expert Q&A

Question

How do you find the x-intercept in y = mx + b?

David Jia
Academic Tutor
David Jia is an Academic Tutor and the Founder of LA Math Tutoring, a private tutoring company based in Los Angeles, California. With over 10 years of teaching experience, David works with students of all ages and grades in various subjects, as well as college admissions counseling and test preparation for the SAT, ACT, ISEE, and more. After attaining a perfect 800 math score and a 690 English score on the SAT, David was awarded the Dickinson Scholarship from the University of Miami, where he graduated with a Bachelor’s degree in Business Administration. Additionally, David has worked as an instructor for online videos for textbook companies such as Larson Texts, Big Ideas Learning, and Big Ideas Math.

David Jia

Academic Tutor

Expert Answer

Find the x-intercept by plugging in 0 for the value of y. The x-intercept will equal whatever value comes out at the other end of the equation.
Question

What do you mean by x-intercept in math?

David Jia
Academic Tutor
David Jia is an Academic Tutor and the Founder of LA Math Tutoring, a private tutoring company based in Los Angeles, California. With over 10 years of teaching experience, David works with students of all ages and grades in various subjects, as well as college admissions counseling and test preparation for the SAT, ACT, ISEE, and more. After attaining a perfect 800 math score and a 690 English score on the SAT, David was awarded the Dickinson Scholarship from the University of Miami, where he graduated with a Bachelor’s degree in Business Administration. Additionally, David has worked as an instructor for online videos for textbook companies such as Larson Texts, Big Ideas Learning, and Big Ideas Math.

David Jia

Academic Tutor

Expert Answer

The x-intercept is just wherever the graph crosses the x-axis (the straight, horizontal line that cuts through the middle of the graph).
Question

If I have (3, -12) as my points and an x-intercept of one, what would the y-intercept be?

Donagan

Top Answerer

Solve by using Method 2 above. You need two points to use that method. One point is given as (3,-12). The second point is the given x-intercept, which is (1,0).