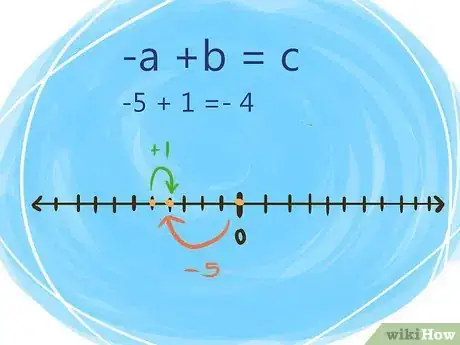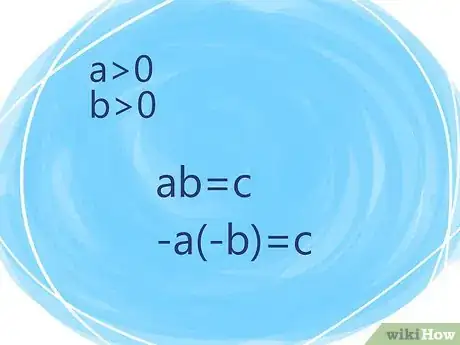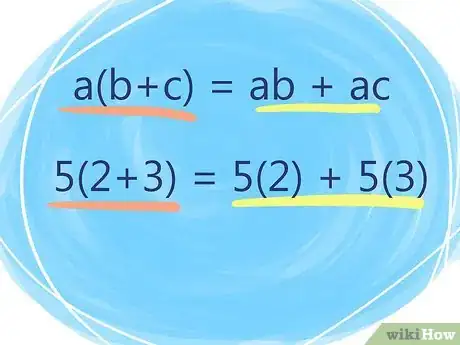wikiHow is a “wiki,” similar to Wikipedia, which means that many of our articles are co-written by multiple authors. To create this article, volunteer authors worked to edit and improve it over time.
This article has been viewed 22,372 times.
Learn more...
An integer is a set of natural numbers, their negatives, and zero. However, some integers are natural numbers, including 1, 2, 3, and so on. Their negative values are, -1, -2, -3, and so on. So integers are the set of numbers including (…-3, -2, -1, 0, 1, 2, 3,…). An integer is never a fraction, decimal, or percentage, it can only be a whole number. To solve integers and use their properties, learn to use addition and subtraction properties and use multiplication properties.
Steps
Using Addition and Subtraction Properties
-
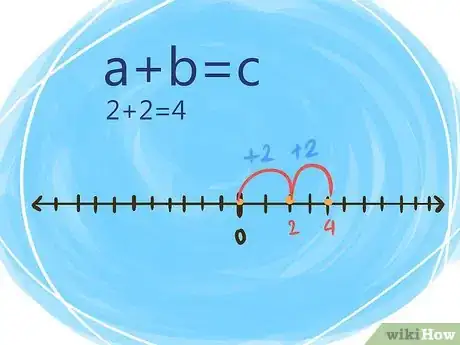
1Use the commutative property when both numbers are positive. The commutative property of addition states that changing the orders of the numbers doesn’t affect the sum of the equation. Do the addition as follows:
- a + b = c (where both a and b are positive numbers the sum c is also positive)
- For example: 2 + 2 = 4
-
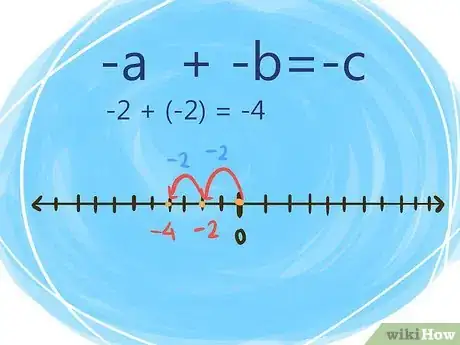
2Use the commutative property if a and b are both negative. Do the addition as follows:
- -a + -b = -c (where both a and b are negative, you get the absolute value of the numbers then you proceed to add, and use the negative sign for the sum)
- For example: -2+ (-2)=-4
Advertisement -
3Use the commutative property when one number is positive and the other is negative. Do the addition as follows:
- a + (-b) = c (when your terms are of different signs, determine the larger number's value, then get the absolute value of both terms and subtract the lesser value from the larger value. Use the sign of the larger number for the answer.)
- For example: 5 + (-1) = 4
-
4Use the commutative property when a is negative and b is positive. Do the addition as follows:
- -a +b = c (get the absolute value of the numbers and again, proceed to subtract the lesser value from the larger value and assume the sign of the larger value)
- For example: -5 + 2 = -3
-
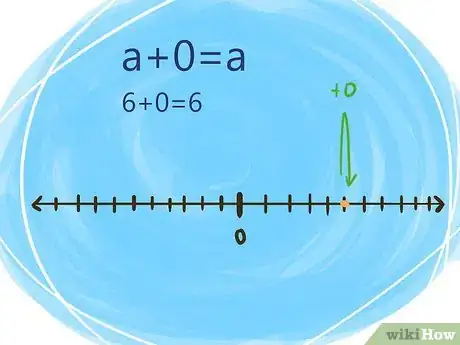
5Understand the additive identity when adding a number to zero. The sum of any number when added to zero, is the number itself.
- An example of the additive identity is: a + 0 = a
- Mathematically, the additive identity looks like: 2 + 0 = 2 or 6 + 0 = 6
-
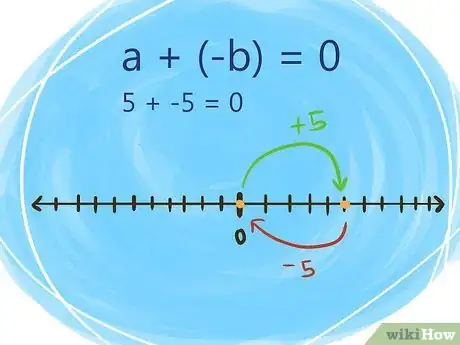
6Know that adding the additive inverse is equal to zero. When adding the additive inverse of a number, the sum is equal to zero.
- The additive inverse is when a number is added to the negative equivalent of itself.
- For example: a + (-b) = 0, where b is equal to a
- Mathematically, the additive inverse looks like: 5 + -5 = 0
-
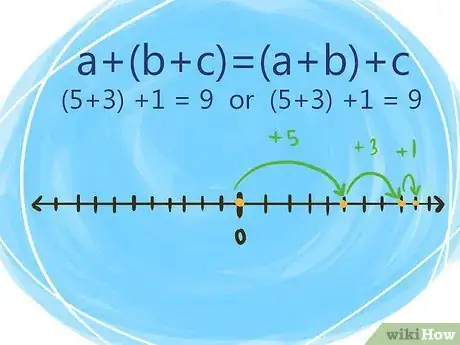
7Realize that the associative property says that regrouping the addends (added numbers) doesn’t change the sum of the equation. The order in which you add numbers does not affect their sum.
- For example: (5+3) +1 = 9 has the same sum as 5+ (3+1) = 9
Using Multiplication Properties
-
1Realize that the associative property of multiplication means that the order in which you multiply does not affect the product of the equation. Multiplying a*b = c is also the same as b*a=c. However, the sign of the product can change depending on the signs of the original numbers:
- If both a and b have the same signs, the sign of the product is positive. For example:
- When a and b are positive numbers and not equal to zero: +a * + b = +c
- When a and b are both negative numbers and not equal to zero: -a*-b = +c
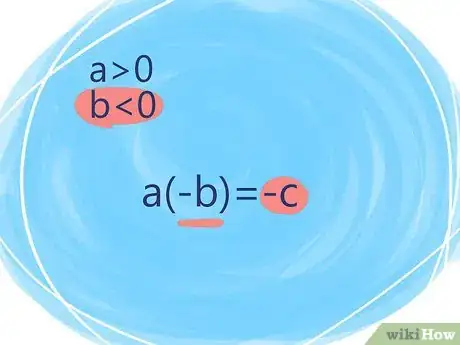
- If a and b have unlike signs, the sign of the product is negative. For example:
- When a is positive and b is negative: +a * -b = -c
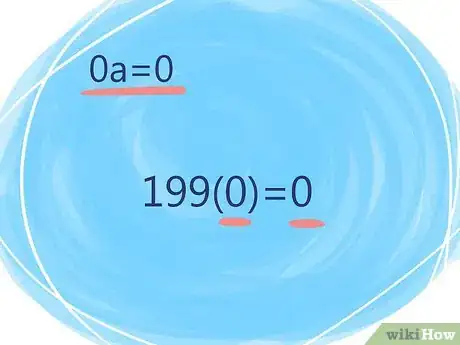
- However, understand that any number multiplied by zero, equals zero.
- If both a and b have the same signs, the sign of the product is positive. For example:
-
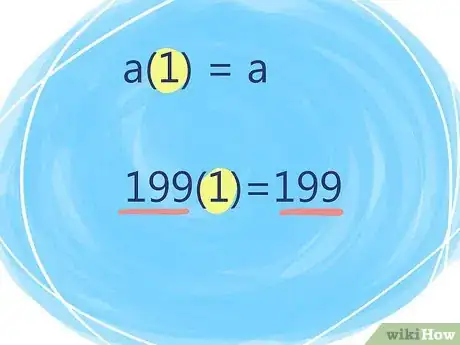
2
-
3Recognize the distributive property of multiplication. The distributive property of multiplication says that any number "a" multiplied by the addends "b" and "c" in parenthesis, is the same as "a" multiplied by "c" plus "a" multiplied by "b".
- For example: a(b+c) = ab + ac
- Mathematically, this looks like: 5(2+3) = 5(2) + 5(3)
- Note that there is no inverse property for multiplication because the inverse of a whole number is a fraction, and fractions are not an element of integer.